Разделы презентаций
- Разное
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Lect 1.ppt
Содержание
- 1. Lect 1.ppt
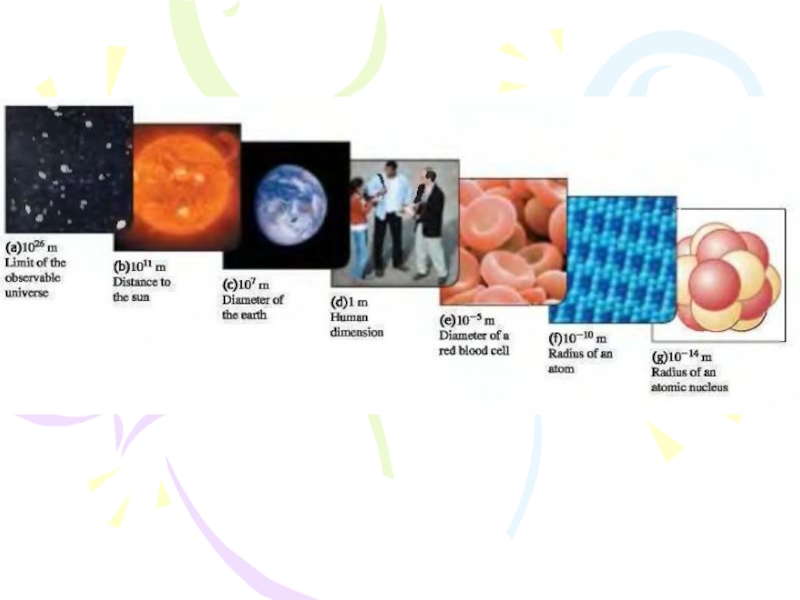
- 2. Various examples of physical phenomena
- 3. Physics (from Ancient Greek: φύσις physis "nature") is a
- 4. Слайд 4
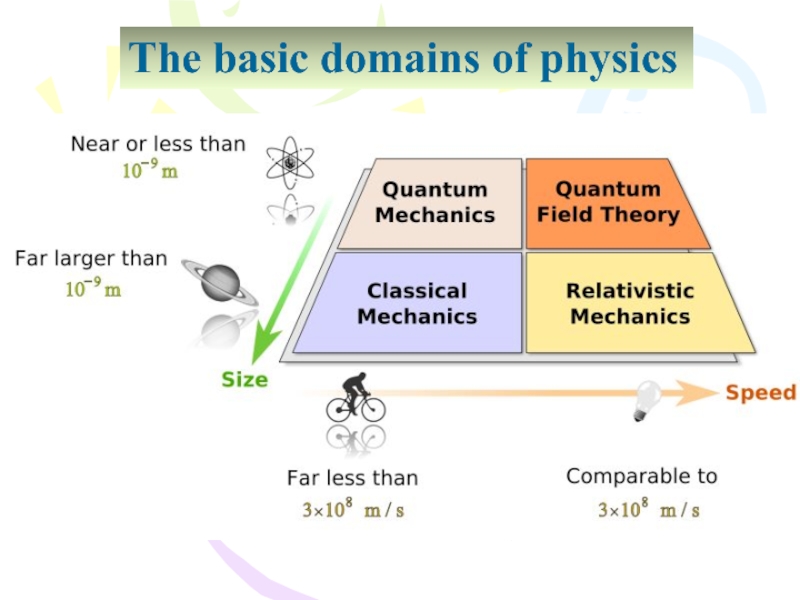
- 5. The basic domains of physics
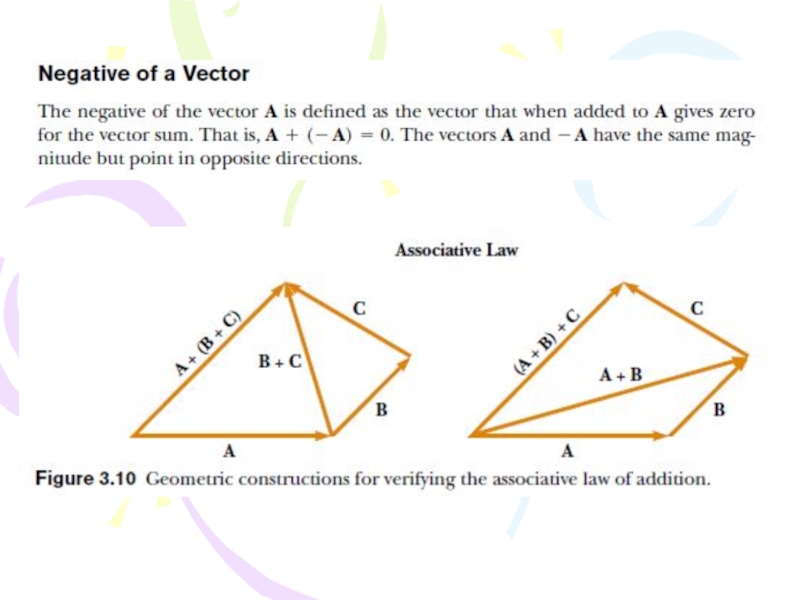
- 6. Galileo Galilei (1564–1642)History of physicsAristotle (384–322 BCE)
- 7. Isaac Newton (1643–1727)Michael Faraday (1791–1867)
- 8. Albert Einstein (1879–1955)Clausius (1822-1888)
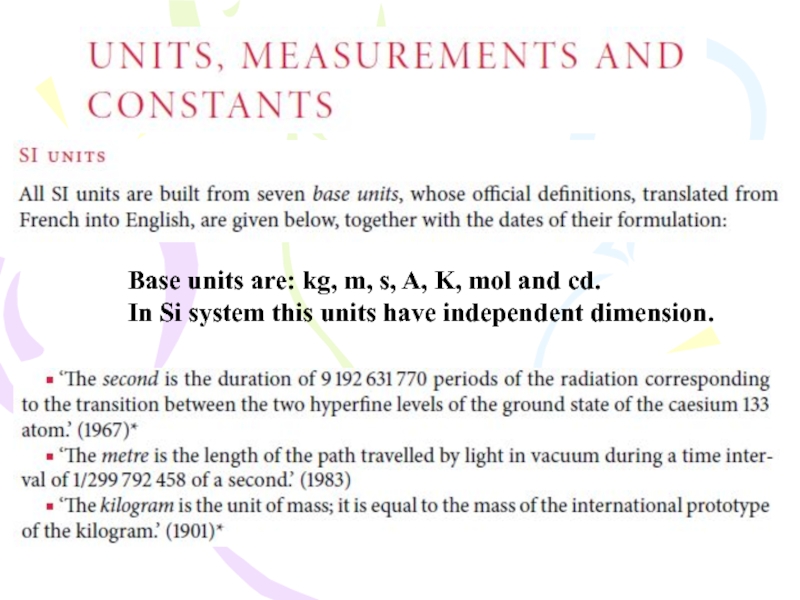
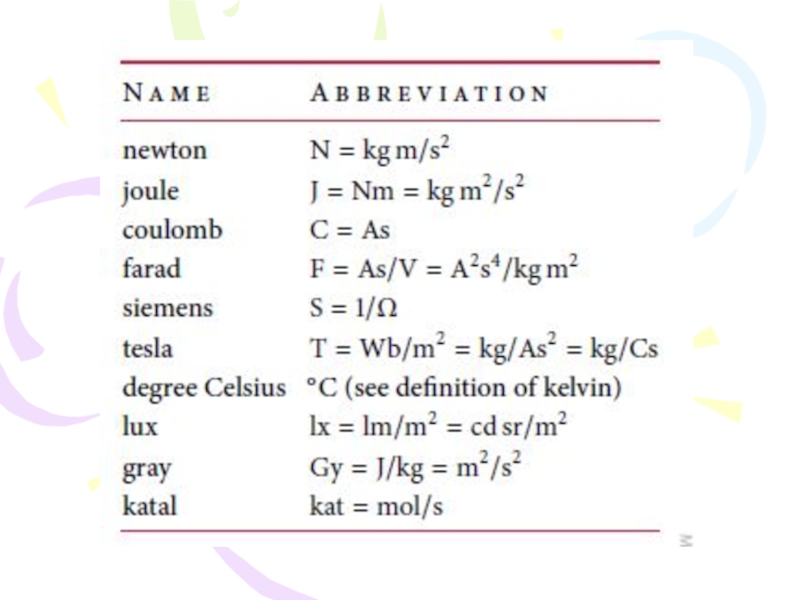
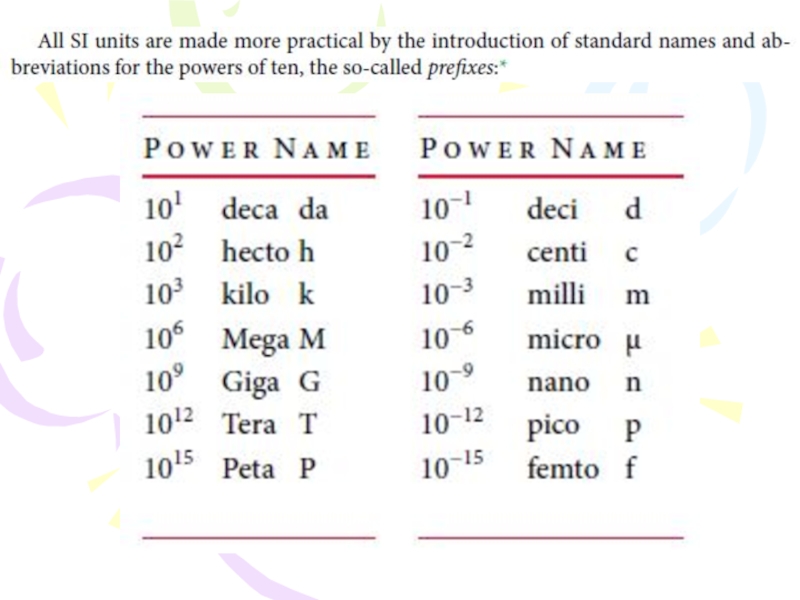
- 9. Base units are: kg, m, s, A,
- 10. Слайд 10
- 11. Слайд 11
- 12. Слайд 12
- 13. Слайд 13
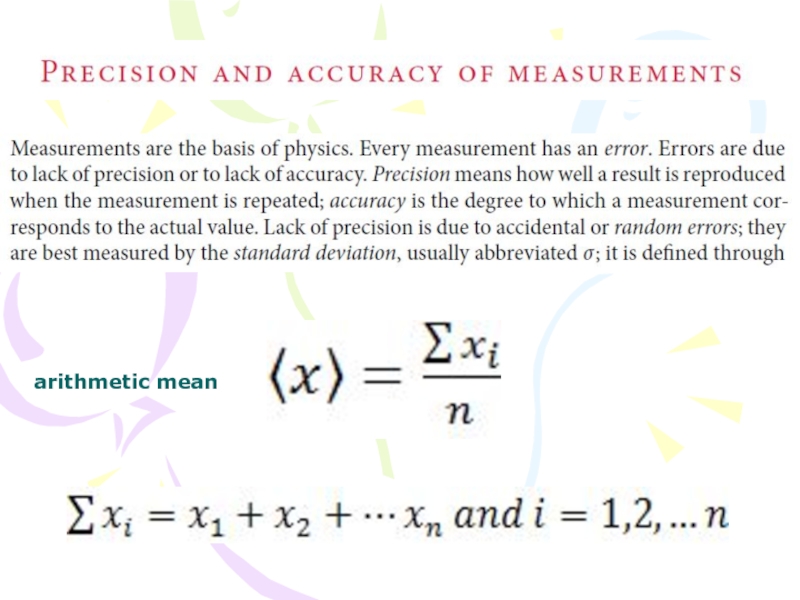
- 14. arithmetic mean
- 15. Absolute error and relative error
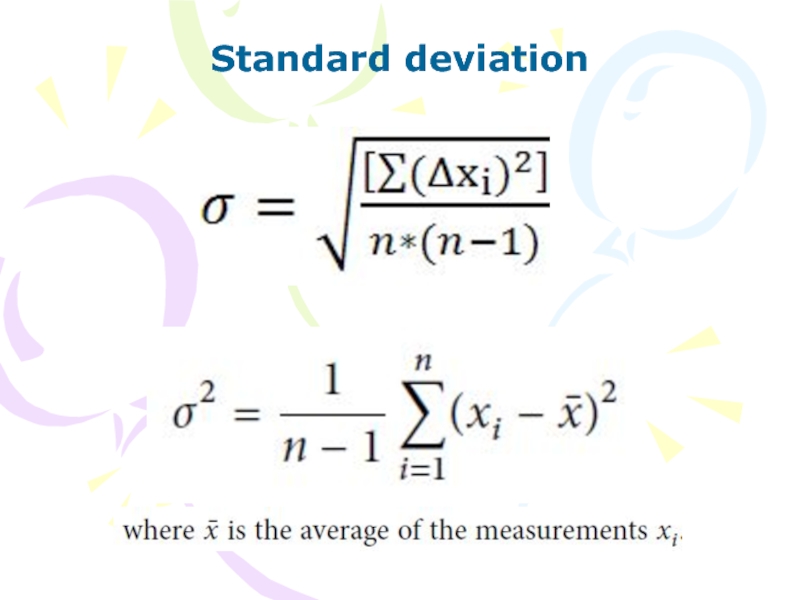
- 16. Standard deviation
- 17. Слайд 17
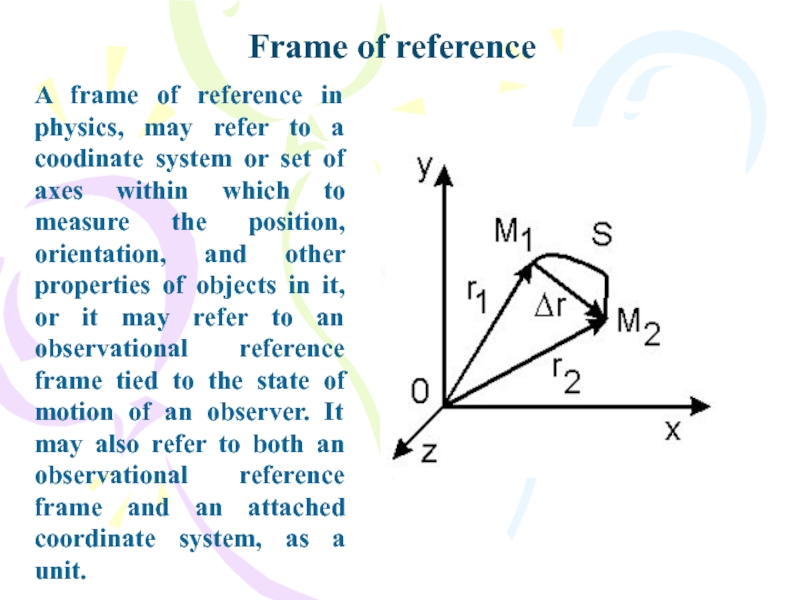
- 18. A frame of reference in physics, may
- 19. Слайд 19
- 20. Слайд 20
- 21. Слайд 21
- 22. Слайд 22
- 23. Dot productThe dot product of two vectors
- 24. Cross productThe cross product (also called the
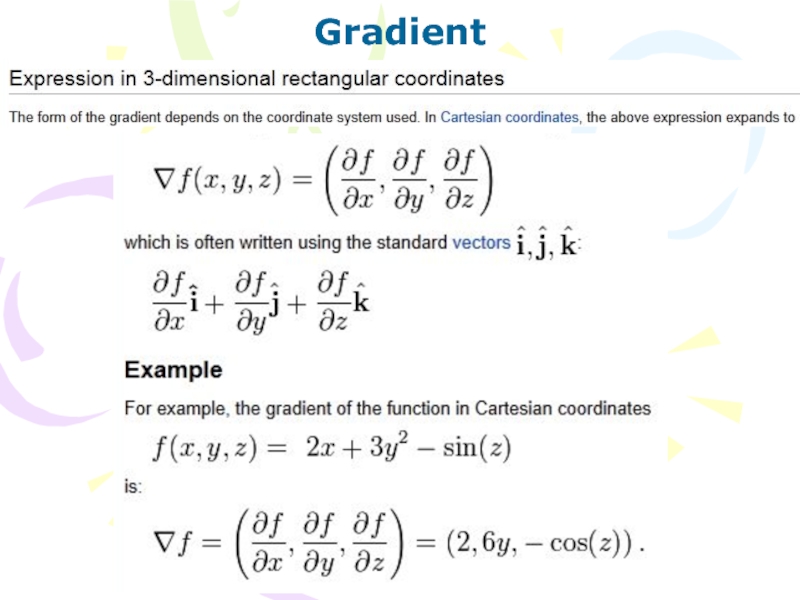
- 25. Gradient
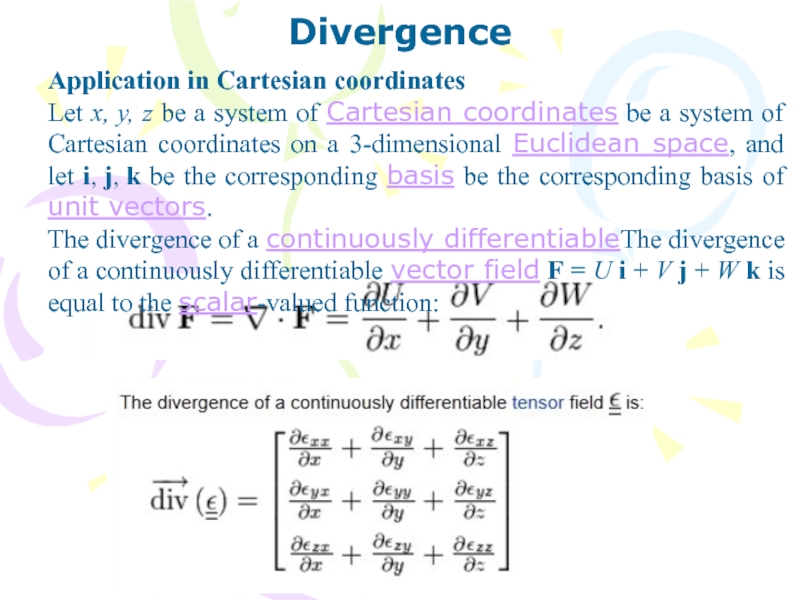
- 26. DivergenceApplication in Cartesian coordinatesLet x, y, z
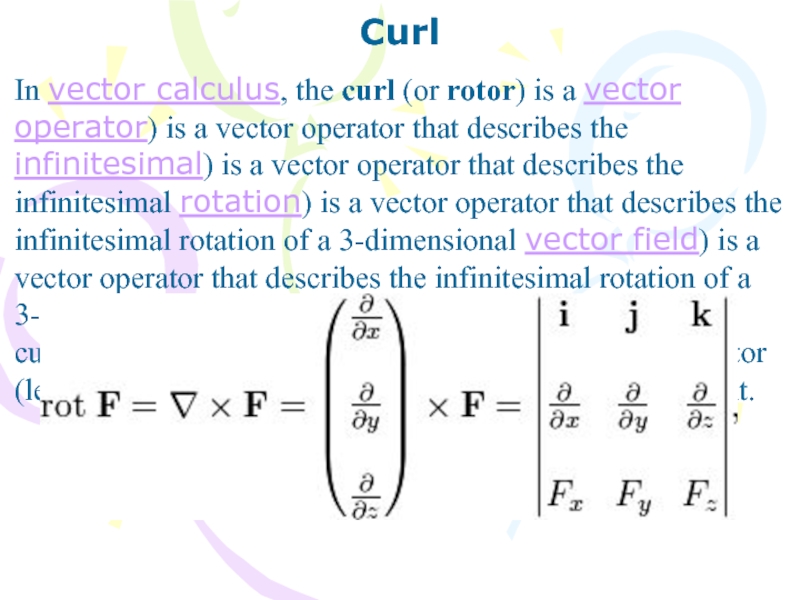
- 27. CurlIn vector calculus, the curl (or rotor)
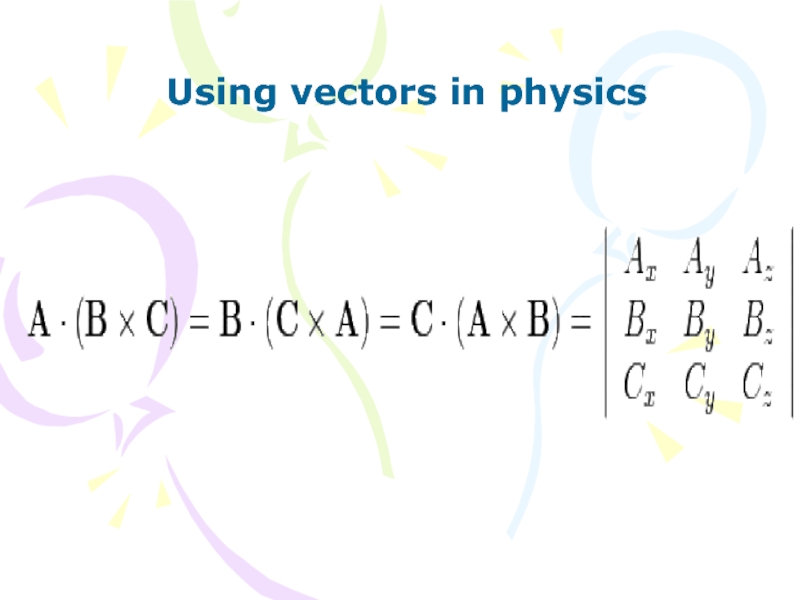
- 28. Using vectors in physics
- 29. Скачать презентанцию
Various examples of physical phenomena
Слайды и текст этой презентации
Слайд 9Base units are: kg, m, s, A, K, mol and
cd.
In Si system this units have independent dimension.
Слайд 18A frame of reference in physics, may refer to a
coodinate system or set of axes within which to measure
the position, orientation, and other properties of objects in it, or it may refer to an observational reference frame tied to the state of motion of an observer. It may also refer to both an observational reference frame and an attached coordinate system, as a unit.Frame of reference
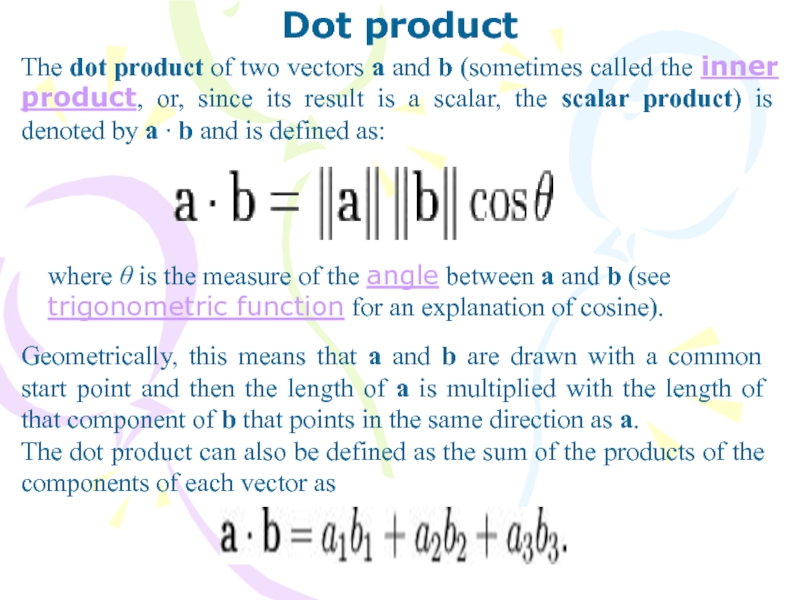
Слайд 23Dot product
The dot product of two vectors a and b
(sometimes called the inner product, or, since its result is
a scalar, the scalar product) is denoted by a ∙ b and is defined as:where θ is the measure of the angle between a and b (see trigonometric function for an explanation of cosine).
Geometrically, this means that a and b are drawn with a common start point and then the length of a is multiplied with the length of that component of b that points in the same direction as a.
The dot product can also be defined as the sum of the products of the components of each vector as
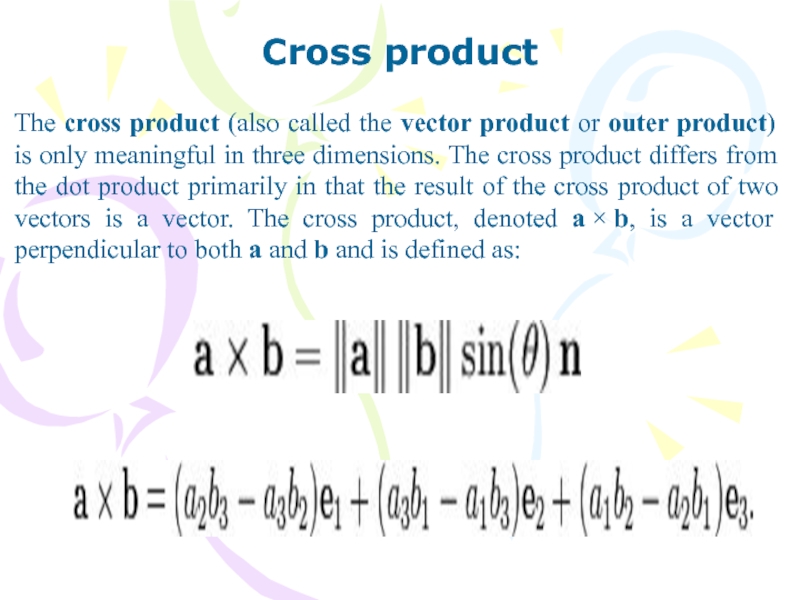
Слайд 24Cross product
The cross product (also called the vector product or
outer product) is only meaningful in three dimensions. The cross
product differs from the dot product primarily in that the result of the cross product of two vectors is a vector. The cross product, denoted a × b, is a vector perpendicular to both a and b and is defined as:
Слайд 26Divergence
Application in Cartesian coordinates
Let x, y, z be a system
of Cartesian coordinates be a system of Cartesian coordinates on
a 3-dimensional Euclidean space, and let i, j, k be the corresponding basis be the corresponding basis of unit vectors.The divergence of a continuously differentiableThe divergence of a continuously differentiable vector field F = U i + V j + W k is equal to the scalar-valued function:
Слайд 27Curl
In vector calculus, the curl (or rotor) is a vector
operator) is a vector operator that describes the infinitesimal) is
a vector operator that describes the infinitesimal rotation) is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field) is a vector operator that describes the infinitesimal rotation of a 3-dimensional vector field. At every point in the field, the curl is represented by a vector. The attributes of this vector (length and direction) characterize the rotation at that point.
