Разделы презентаций
- Разное
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Охватывающий эллипсоид
Содержание
- 1. Охватывающий эллипсоид
- 2. Введение. Постановка задачи.Пусть в пространстве
- 3. Охватывающий эллипсоид10.03.2012
- 4. 10.03.2012Охватывающий эллипсоид
- 5. Охватывающий эллипсоид10.03.2012
- 6. Dual Reduced Newton Algorithm10.03.2012In this section, we
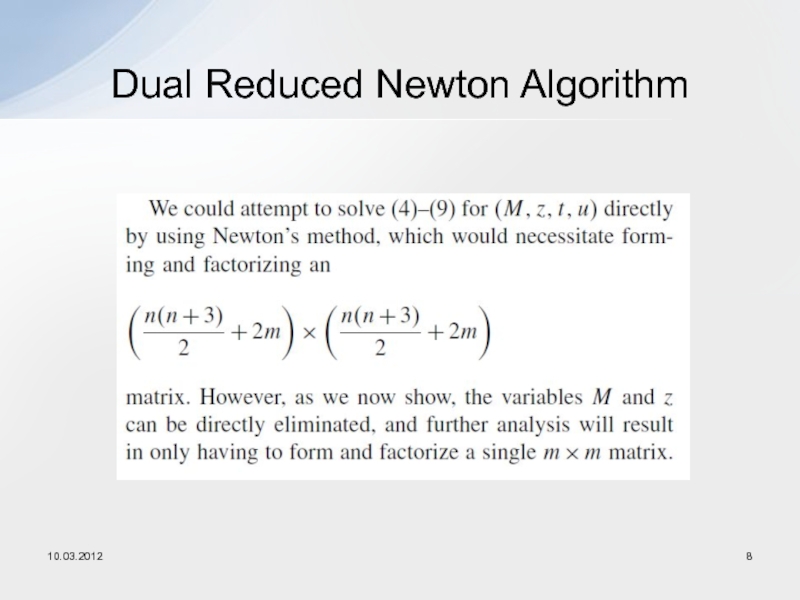
- 7. Dual Reduced Newton Algorithm10.03.2012
- 8. Dual Reduced Newton Algorithm10.03.2012
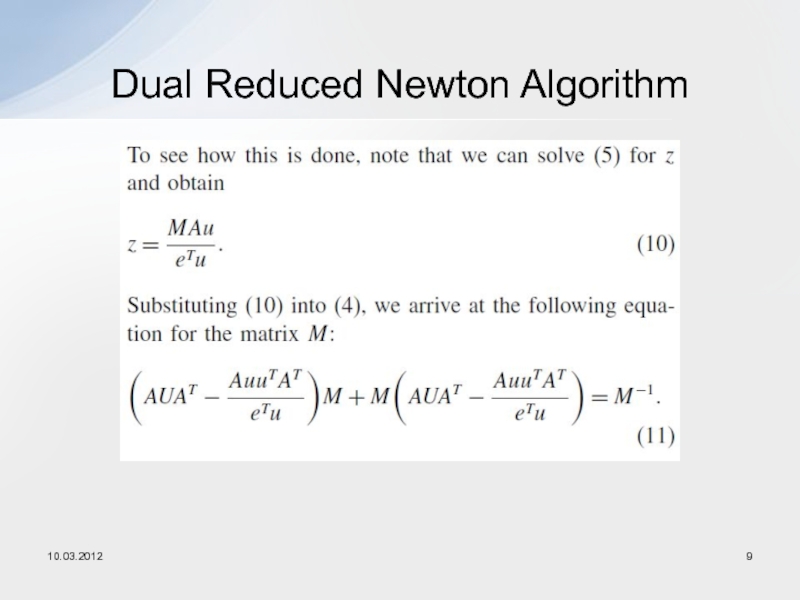
- 9. Dual Reduced Newton Algorithm10.03.2012
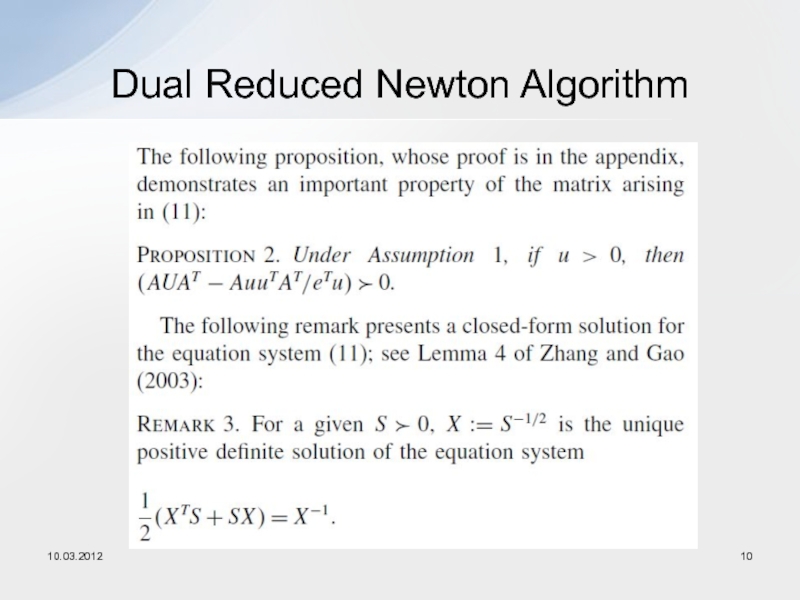
- 10. Dual Reduced Newton Algorithm10.03.2012
- 11. Dual Reduced Newton Algorithm10.03.2012
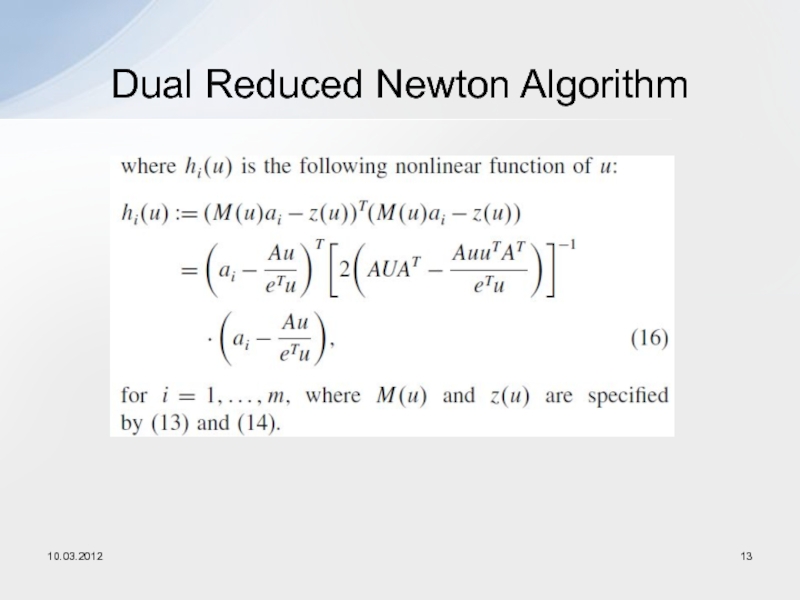
- 12. Dual Reduced Newton Algorithm10.03.2012
- 13. Dual Reduced Newton Algorithm10.03.2012
- 14. Dual Reduced Newton Algorithm10.03.2012
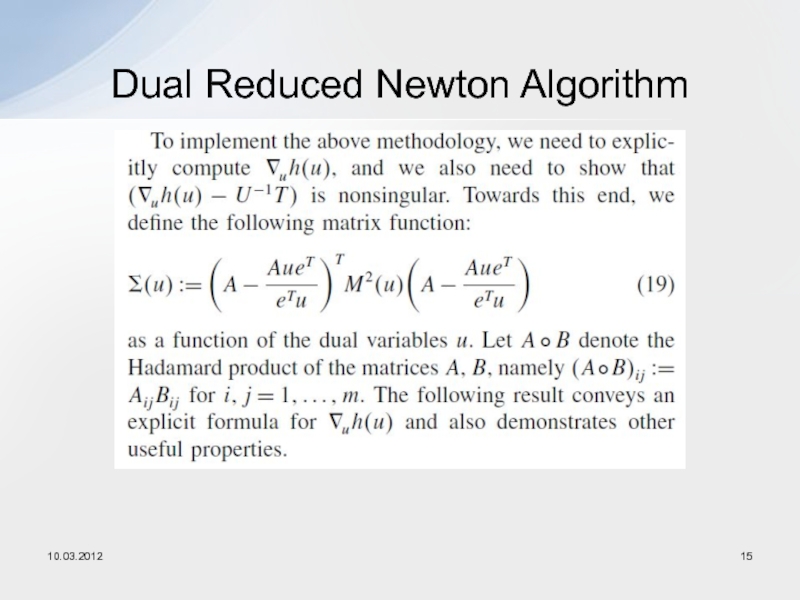
- 15. Dual Reduced Newton Algorithm10.03.2012
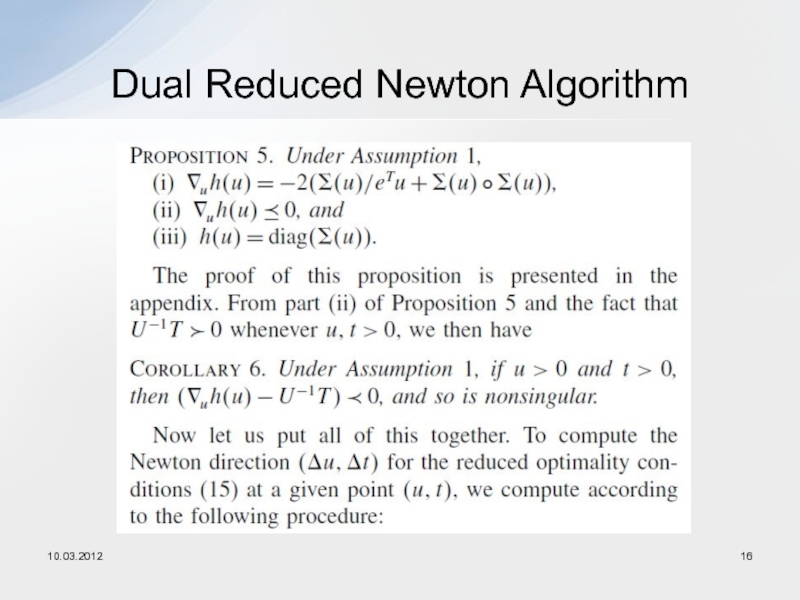
- 16. Dual Reduced Newton Algorithm10.03.2012
- 17. Dual Reduced Newton Algorithm10.03.2012
- 18. Dual Reduced Newton Algorithm10.03.2012
- 19. Dual Reduced Newton Algorithm10.03.2012Based on the Newton
- 20. Dual Reduced Newton Algorithm10.03.2012
- 21. Dual Reduced Newton Algorithm10.03.2012
- 22. Dual Reduced Newton Algorithm10.03.2012
- 23. Dual Reduced Newton Algorithm10.03.2012
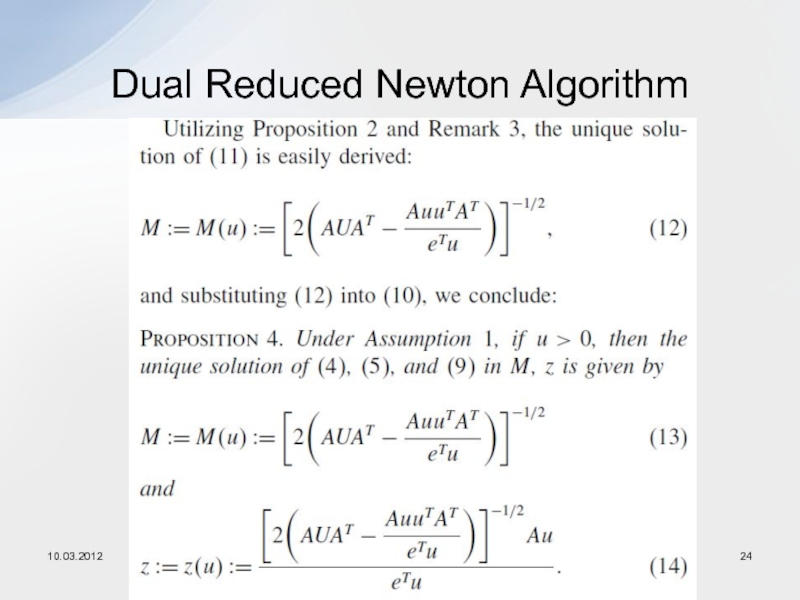
- 24. Dual Reduced Newton Algorithm10.03.2012
- 25. Dual Reduced Newton Algorithm10.03.2012
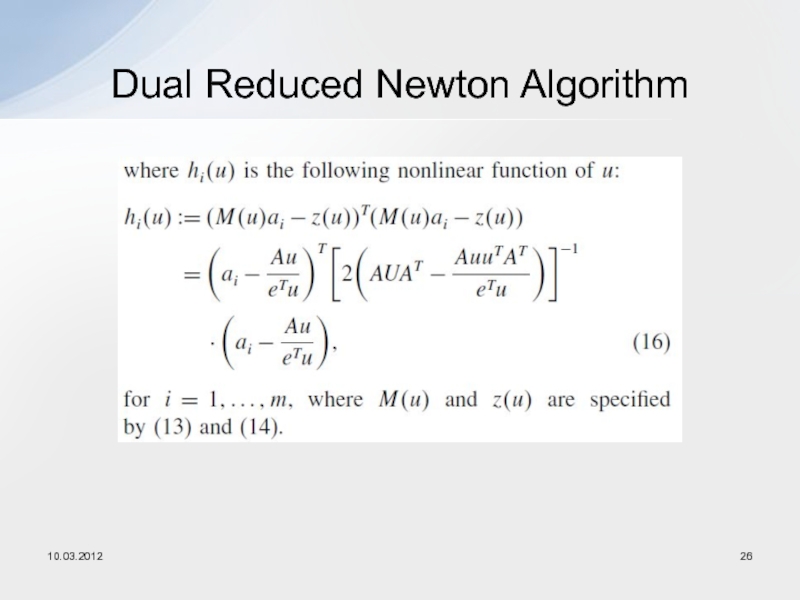
- 26. Dual Reduced Newton Algorithm10.03.2012
- 27. Dual Reduced Newton Algorithm10.03.2012
- 28. Dual Reduced Newton Algorithm10.03.2012
- 29. Dual Reduced Newton Algorithm10.03.2012
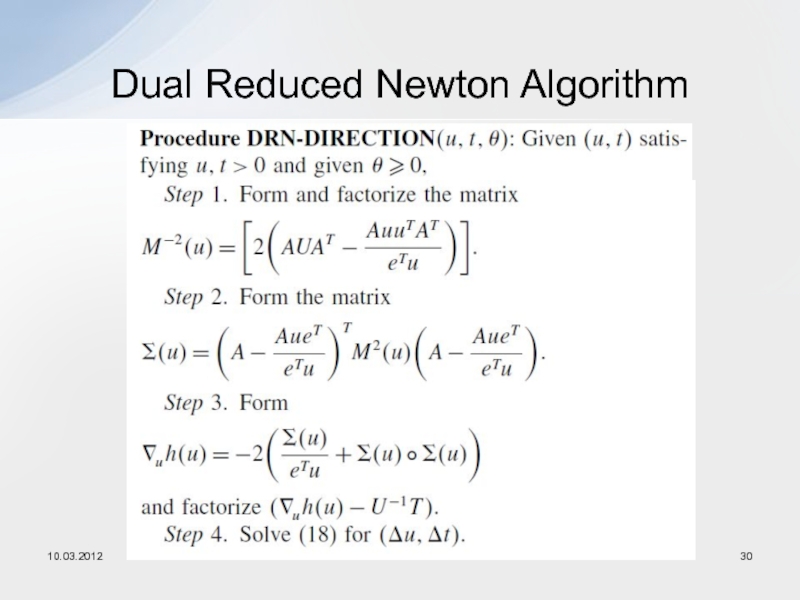
- 30. Dual Reduced Newton Algorithm10.03.2012
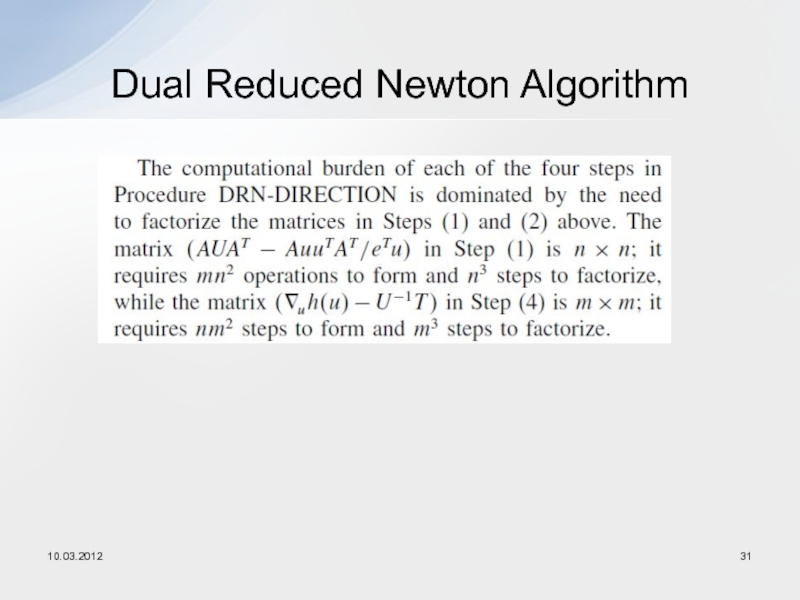
- 31. Dual Reduced Newton Algorithm10.03.2012
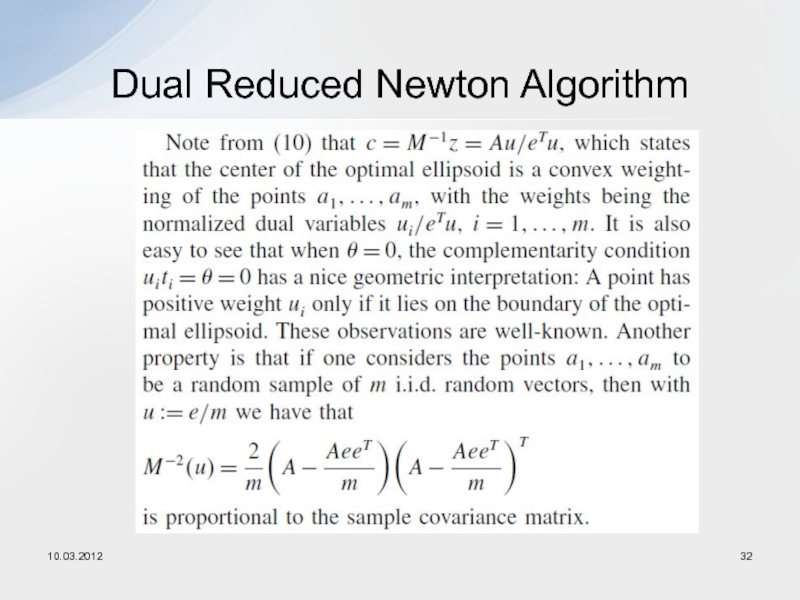
- 32. Dual Reduced Newton Algorithm10.03.2012
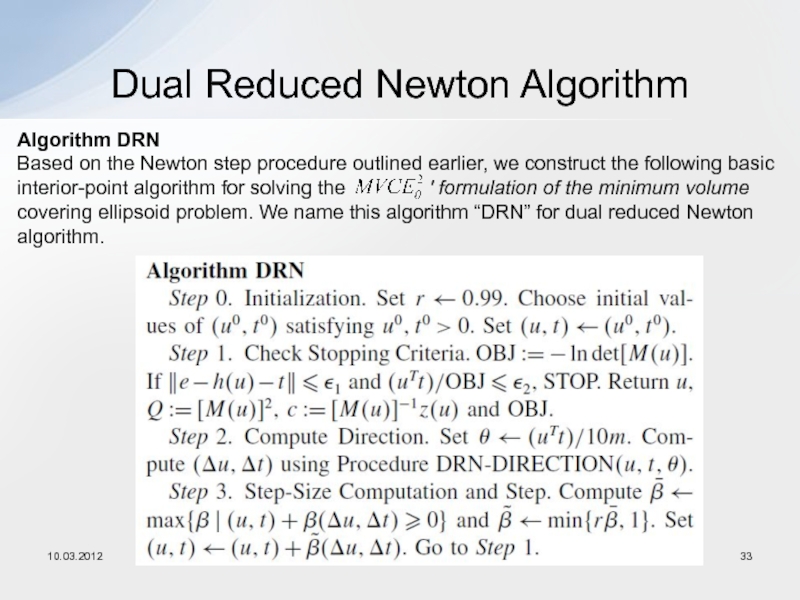
- 33. Dual Reduced Newton Algorithm10.03.2012Algorithm DRNBased on the
- 34. 1. Computation of Minimum-Volume Covering Ellipsoids. Peng
- 35. Скачать презентанцию
Введение. Постановка задачи.Пусть в пространстве даны m точекТребуется построить эллипсоид минимального объема,содержащий внутри себя все эти точки.Обозначим через матрицу размерности ,столбцы которой являются
Слайды и текст этой презентации
Слайд 1ЧИСЛЕННЫЕ МЕТОДЫ ОПТИМИЗАЦИИ
Константин Ловецкий
Ноябрь 2011
Кафедра систем телекоммуникаций
Оптимизация.
Построение минимального
охватывающего
эллипсоида
Слайд 2Введение. Постановка задачи.
Пусть в пространстве даны m точек
Требуется
построить эллипсоид минимального объема,
содержащий внутри себя все эти точки.
Обозначим через
матрицу размерности ,столбцы которой являются векторами .
Определение эллипсоида:
Эллипсоид с центром в точке определяется как
Охватывающий эллипсоид
10.03.2012
Слайд 6Dual Reduced Newton Algorithm
10.03.2012
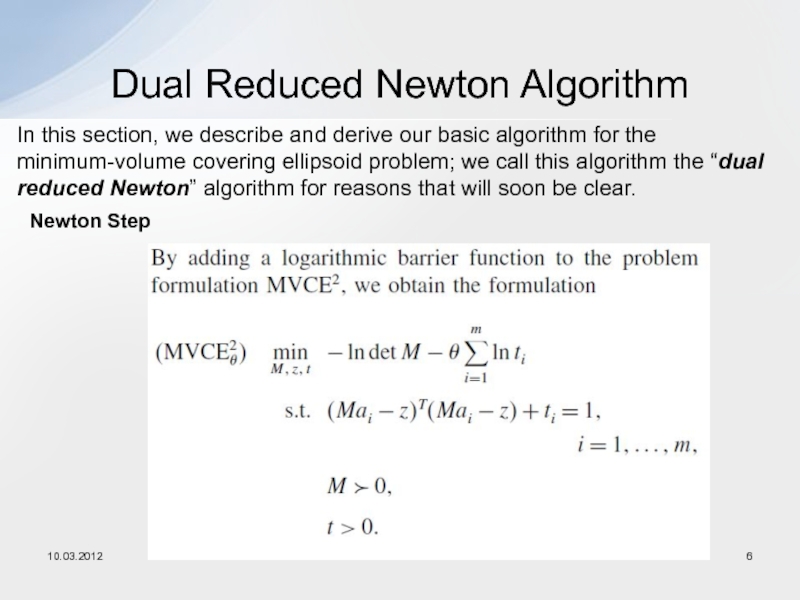
In this section, we describe and derive
our basic algorithm for the minimum-volume covering ellipsoid problem; we
call this algorithm the “dual reduced Newton” algorithm for reasons that will soon be clear.Newton Step
Слайд 19Dual Reduced Newton Algorithm
10.03.2012
Based on the Newton step procedure outlined
ealier, we construct the following basic interior-point algorithm for solving
the MVCE 2 ‘formulation of the minimum volume’covering ellipsoid problem.
We name this algorithm “DRN” for dual reduced Newton algorithm.
Newton Step
Слайд 33Dual Reduced Newton Algorithm
10.03.2012
Algorithm DRN
Based on the Newton step procedure
outlined earlier, we construct the following basic interior-point algorithm for
solving the ' formulation of the minimum volumecovering ellipsoid problem. We name this algorithm “DRN” for dual reduced Newton algorithm.
Слайд 341. Computation of Minimum-Volume Covering Ellipsoids. Peng Sun, Robert M.
Freund. OPERATIONS RESEARCH Vol. 52, No. 5, September–October 2004, pp.
690–706Литература
10.03.2012