Слайд 1Толық квадрат
теңдеулерді шешу
Full quadratic equations
Слайд 2
New words:
Equation - теңдеу
simple equation – сызықтық теңдеу
equivalent equation- мәндес
теңдеулер
Root - түбір
properties of a quadratic equation – квадрат түбірдің
қасиеттері
sum square – екі өрнектің қосындысының
difference of two expressions- айырымының квадраты
real numbers – нақты сандар
A – the first coefficient – бірінші коэффициент
B – the second coefficient- екінші коэффициент
C - constant term – бос мүше
Graph - граф
Parabola – парабола
IF- мұндағы
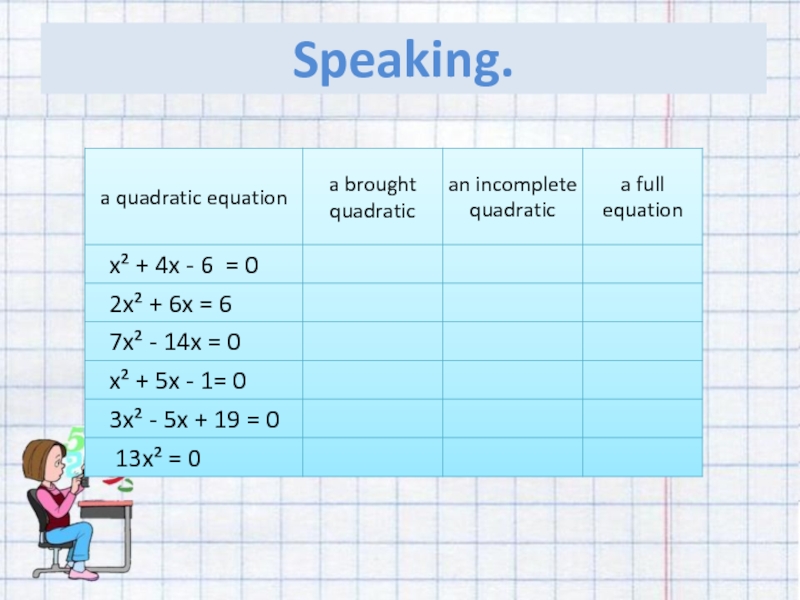
Слайд 3A quadratic equation is an equation that can be written
in the form ax2+bx+c=0, where a,b and c are real
numbers and a ≠0
A – the first coefficient
B – the second coefficient
C - constant term
If in the equation b≠0,c≠0 , then it is called a full equation (толық квадрат теңдеу)
If in a full quadratic equation a=1, it is called brought quadratic the equation (келтірілген квадрат теңдеу)
If b or c, or both are equal to zero, then the equation is called as an incomplete quadratic equation
Слайд 4 2000 years ago in Babylon people кnew how to solve
quadratic equations
Their solution in Ancient Babylon was closely connected with
practical problems, mainly such as the measurement of land, land work related to military needs; the presence of these knowledge is also due to the development of mathematics and astronomy in general. There have been known methods of solving both full and incomplete quadratic equations. We give examples of quadratic equations, is solved in ancient Babylon, using modern algebraic entry
Слайд 5India
Problems solved using quadratic equations found in a treatise
on astronomy "Ariabhattiam" written by an Indian astronomer and mathematician
Aryabhata in 499 AD. One of the first conclusions of the formula known roots of a quadratic equation belongs to an Indian scientist Brahmagupta (about 598 g.) [1]; Brahmagupta stated universal rule solving a quadratic equation, the given to the canonical form: ax ^ 2 + bx = c; moreover, it was assumed that all the coefficients in it than a, may be negative. Scientists formulated a rule in essence the same as the modern.
Слайд 8Бір есепті әр түрлі тәсілмен шығару.
х²-4х+3=0 теңдеуін шешіңдер.
1-топ. Көбейткіштерге жіктеу
тәсілімен
2-топ. Екімүшенің квадратын айырып алу әдісімен
3-топ. Формула арқылы шешу тәсілімен
4-топ.басқа
әдістермен
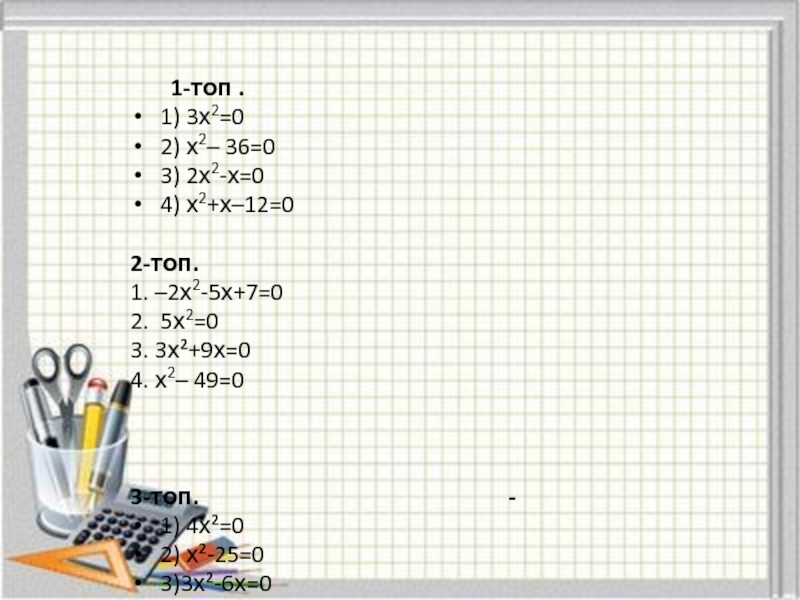
Слайд 10 1-топ .
1) 3х2=0
2) х2– 36=0
3) 2х2-х=0
4) х2+х–12=0
2-топ.
1.
–2х2-5х+7=0
2. 5х2=0
3. 3х²+9х=0
4. х2– 49=0
3-топ. -
1) 4х²=0
2) х²-25=0
3)3х²-6х=0
4)8х²-4х+5=0
4-топ.
1) -2х²=0
2) х²-64=0
3) 2у+у²=0
4)х²+6х-19=0
Слайд 11 1-топ .
1) 0
2) Х1=6; Х2=-6
3) Х1=0; Х2=0,5
4) Х1=-4; Х2=3
2-топ.
1) Х1=1; Х2=-3,5
2) Х=0
3) Х1=0;
Х2=-3
4) Х1=-7; Х2=7
3-топ. -
1) 0
2) Х1=5; Х2=-5
3) Х1=0; Х2=2
4) шешімі жоқ
4-топ.
1) 0
2) Х1=8; Х2=-8
3) Х1=0; Х2=2
4) х=-3
Слайд 12I нұсқа
1. Теңдеуді шешіңіз: (5х-2)(3х+2)=0
А. 5;3
В. С. 2,5 ; 105 D
.
2. Еге р a,b,c-ның а=5, b=2, с= -3-ның мәндері белгілі болса, онда ax2+bx+c=0 теңдеуін құрындар:
А. -5х2-2х+3=0; B. 5х2-2х+3=0; С. 5х2+2х-3=0; D. х2+2х-3=0
3. 5х2-20=0
А. Х1=2; Х1=-2; В. Х1=4; Х1=-4; С. Х1=0; Х1=2; D.Х1=2; Х1=4;
4. 3х2-2х-1=0 теңдеуіндегі а-ның мәні неге тең?
А. 2; В. 3; С. -3; D. 1;
5. Келтірілген теңдеудегі а-ның мәні неге тең?
А. 0; В. 2; С. -1; D. 1;
II нұсқа
Теңдеуді шешіңіз: (2х+3)(6-3х)=0
А. -1,5; 2 В. 1;0 С. 3;6 D. 1,2;2
2. Егер a,b,c-ның а=3, b=-2, с= 1-ның мәндері белгілі болса, онда ax2+bx+c=0 теңдеуән құрындар:
А. х2-2х+1=0; B. 3х2-2х-1=0; С. -3х2+2х-1=0; D. 3х2-2х+1=0
3. 2х2-8=0
А. Х1=4; Х1=-4; В. Х1=2; Х1=-2; С. Х1=0; Х1=2; D.Х1=2; Х1=4;
4. 6х2-5х-1=0 теңдеуіндегі b -ның мәні неге тең?
А. 2; В. 5; С. -5; D. 1;
5. Квадрат теңдеудің дәрежесі неге тең?
А. 0; В. 1; С. -1; D. 2;
Слайд 13Дұрыс жауаптары
І нұсқа
1. В
2. С
3. А
4. В
5. Д
ІІ нұсқа
1. А
2.
Д
3. В
4. С
5. Д