Слайд 1Chapter 3
Understanding the Time Value of Money
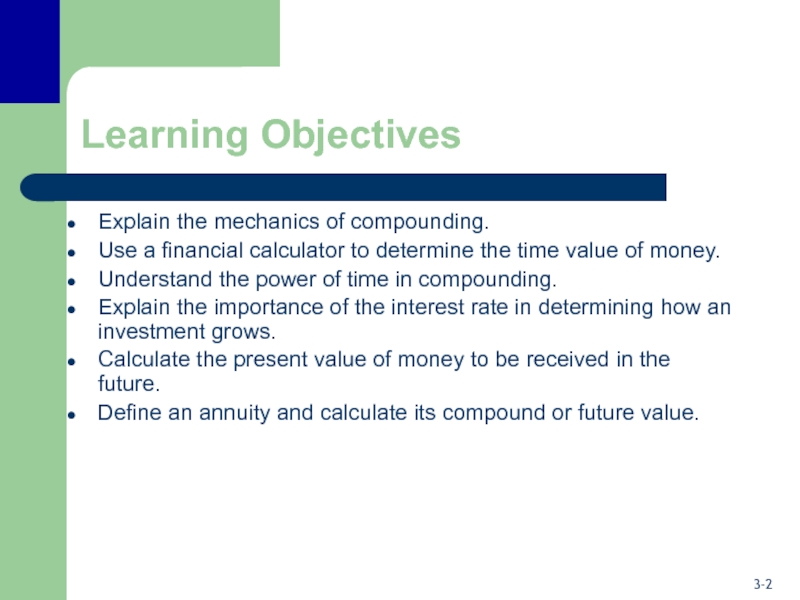
Слайд 2Learning Objectives
Explain the mechanics of compounding.
Use a financial calculator to
determine the time value of money.
Understand the power of time
in compounding.
Explain the importance of the interest rate in determining how an investment grows.
Calculate the present value of money to be received in the future.
Define an annuity and calculate its compound or future value.
Слайд 3Compound Interest and
Future Values
Compound interest is interest on interest.
If you
take interest earned on an investment and reinvest it, you
earn interest on the principal and the reinvested interest.
The amount of interest grows, or compounds.
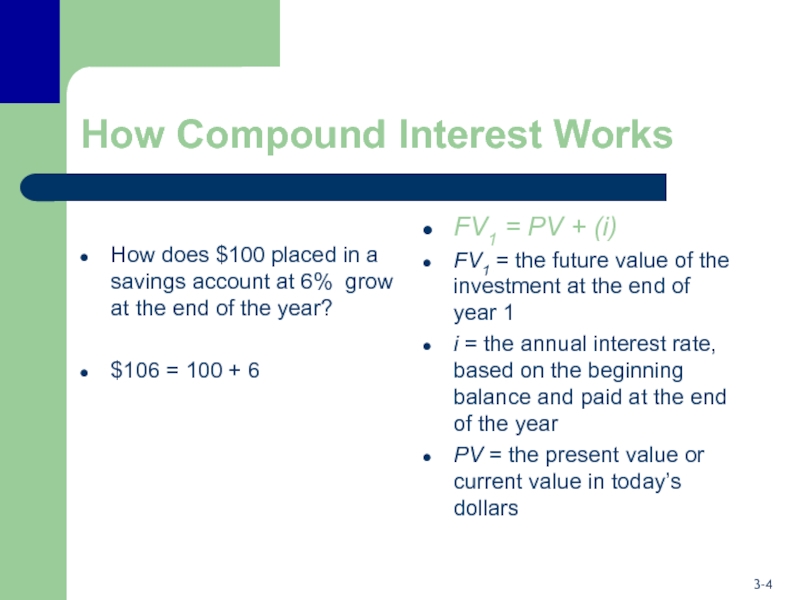
Слайд 4How Compound Interest Works
How does $100 placed in a savings
account at 6% grow at the end of the year?
$106 = 100 + 6
FV1 = PV + (i)
FV1 = the future value of the investment at the end of year 1
i = the annual interest rate, based on the beginning balance and paid at the end of the year
PV = the present value or current value in today’s dollars
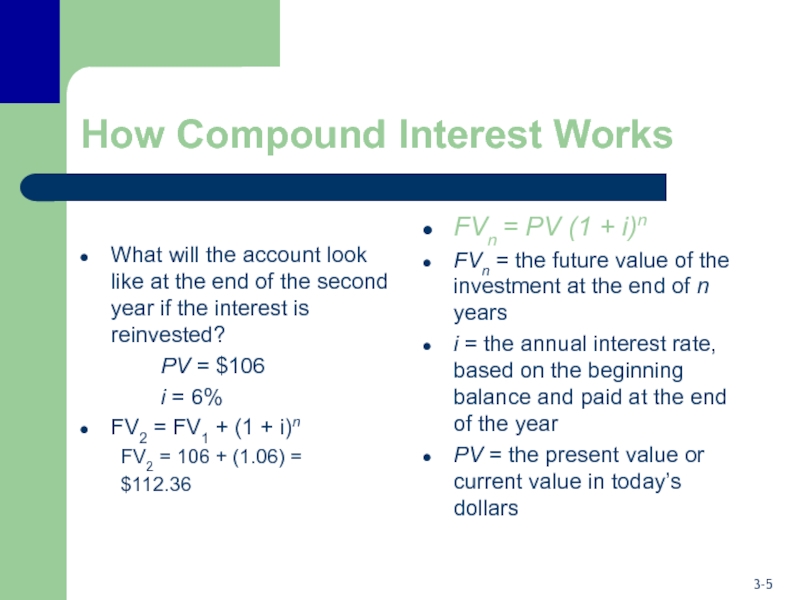
Слайд 5How Compound Interest Works
What will the account look like at
the end of the second year if the interest is
reinvested?
PV = $106
i = 6%
FV2 = FV1 + (1 + i)n
FV2 = 106 + (1.06) =
$112.36
FVn = PV (1 + i)n
FVn = the future value of the investment at the end of n years
i = the annual interest rate, based on the beginning balance and paid at the end of the year
PV = the present value or current value in today’s dollars
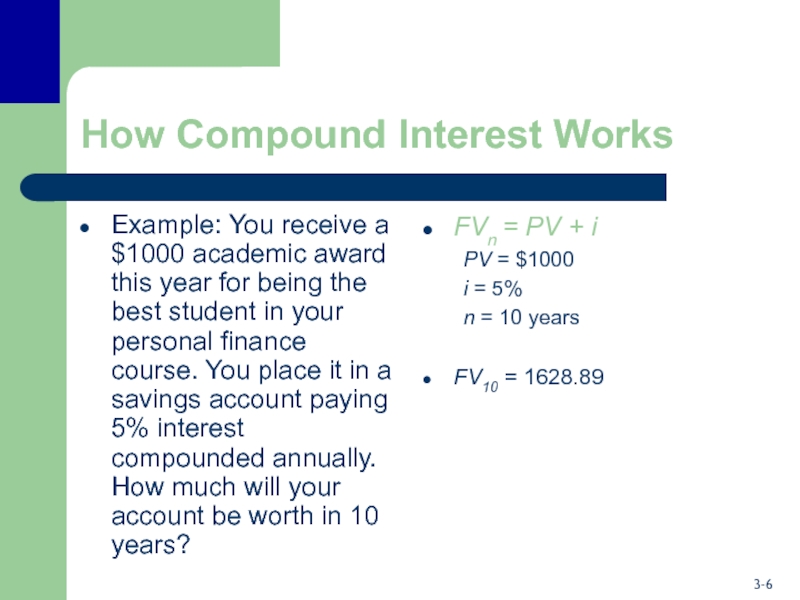
Слайд 6How Compound Interest Works
Example: You receive a $1000 academic award
this year for being the best student in your personal
finance course. You place it in a savings account paying 5% interest compounded annually. How much will your account be worth in 10 years?
FVn = PV + i
PV = $1000
i = 5%
n = 10 years
FV10 = 1628.89
Слайд 7The Future-Value Interest Factor
Calculating future values by hand can be
difficult.
Use a calculator or tables.
The future-value interest factor, found
in a table, replaces the (1 + i)n part of the equation.
Слайд 8The Future-Value Interest Factor
The amounts in the table represent the
value of $1 compounded at rate of i at the
end of nth year.
FVIFi, n is multiplied by the initial investment to calculate the future value of that investment.
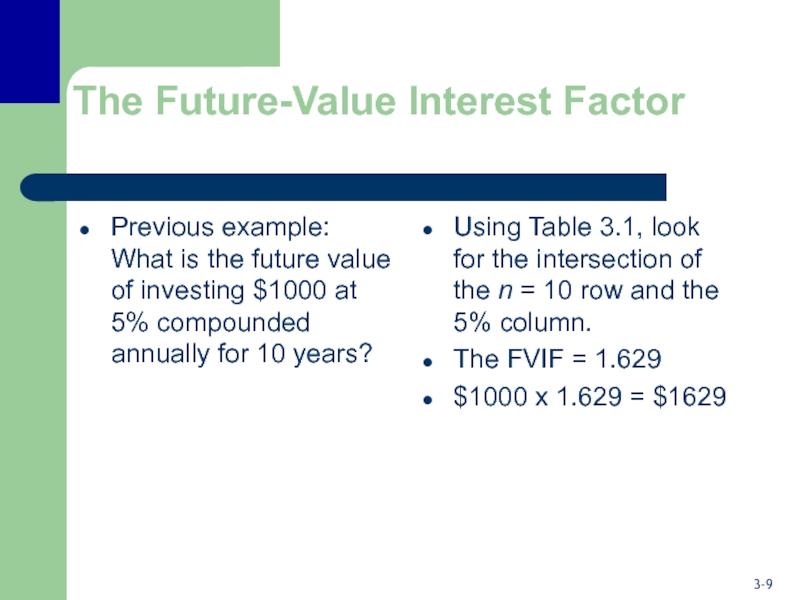
Слайд 9The Future-Value Interest Factor
Previous example: What is the future value
of investing $1000 at 5% compounded annually for 10 years?
Using Table 3.1, look for the intersection of the n = 10 row and the 5% column.
The FVIF = 1.629
$1000 x 1.629 = $1629
Слайд 10The Rule of 72
How long will it take to double
your money?
The Rule of 72 determines how many years
it will take for a sum to double in value by dividing the annual growth or interest rate into 72.
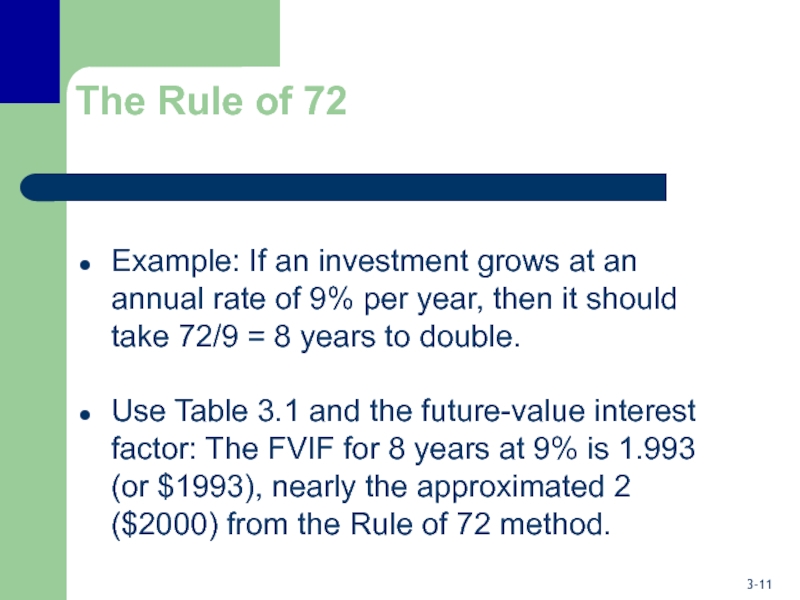
Слайд 11The Rule of 72
Example: If an investment grows at an
annual rate of 9% per year, then it should take
72/9 = 8 years to double.
Use Table 3.1 and the future-value interest factor: The FVIF for 8 years at 9% is 1.993 (or $1993), nearly the approximated 2 ($2000) from the Rule of 72 method.
Слайд 12Compound Interest with Nonannual Periods
Compounding periods may not always be
annually.
Compounding may be quarterly, monthly, daily, or even a
continuous basis.
The sooner interest is paid, the sooner interest is earned on it, and the sooner the benefits or compounding is realized.
Money grows faster as the compounding period becomes shorter.
Слайд 13Compounding and the
Power of Time
Manhattan was purchased in 1626 for
$24 in jewelry and trinkets.
Had that $24 been invested
at 8% compounded annually, it would be worth over $120.6 trillion today.
This illustrates the incredible power of time in compounding.
Слайд 14The Importance of the
Interest Rate
The interest rate plays a critical
role in how much an investment grows.
Consider the “daily
double” where a penny doubles in value each day. By the end of the month, it will grow to over $10 trillion.
Albert Einstein called compound interest “the eighth wonder of the world.”
Слайд 15Present Value
Present value is the value of today’s dollars of
money to be received in the future.
Present value strips
away inflation to see what future cash flows are worth today.
Allows comparisons of dollar values from different periods.
Слайд 16Present Value
Finding present values means moving future money back to
the present.
This is the inverse of compounding.
The “discount rate” is
the interest rate used to bring future money back to present.
Слайд 17Present Value
PV = FVn[1/(1 + i)n]
PV = present value of
a sum of money.
FV = future value of investment at
the end of n years.
n = number of years until payment will be received.
i = annual discount (or interest) rate.
The present value of a future sum of money is inversely related to both the number of years until payment will be received and the discount rate.
Слайд 18Present Value
Tables can be used to calculate the [1/(1+i)n] part
of the equation.
This is the present-value interest factor (PVIF).
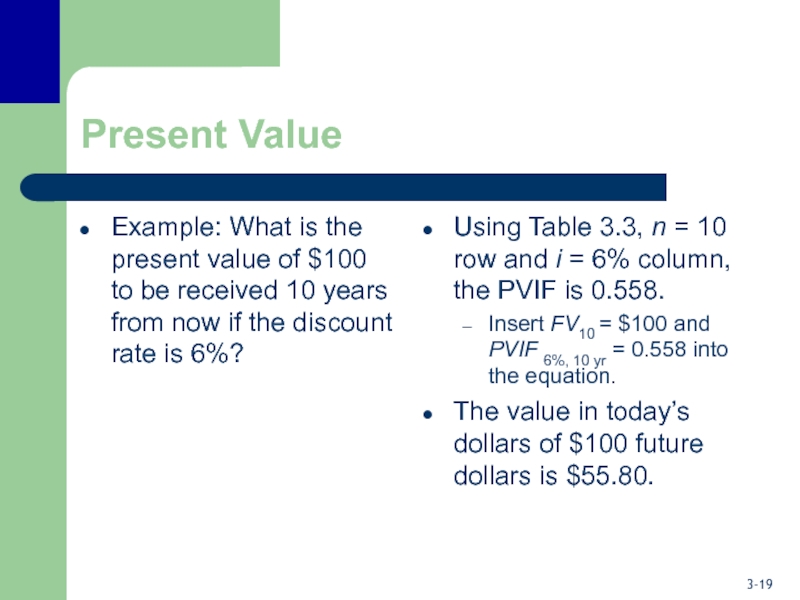
Слайд 19Present Value
Example: What is the present value of $100 to
be received 10 years from now if the discount rate
is 6%?
Using Table 3.3, n = 10 row and i = 6% column, the PVIF is 0.558.
Insert FV10 = $100 and PVIF 6%, 10 yr = 0.558 into the equation.
The value in today’s dollars of $100 future dollars is $55.80.
Слайд 20Present Value
Example: You have been promised $500,000 payable 40 years
from now. What is the value today if the discount
rate is 6%?
PV = FVn(PVIF i%, n yrs)
Using Table 3.3, n = 40 row and i = 6% column, the PVIF is 0.097.
Multiply the $500,000 by 0.097.
The value in today’s dollars is $48,500.
Слайд 21Present Value
You’ve just seen that $500,000 payable 40 years from
now, with a discount rate of 6%, is worth $48,500
in today’s dollars.
Conversely, if you deposit $48,500 in the bank today, earning 6% interest annually, in 40 years you would have $500,000.
There is really only one time value money equation.
Слайд 22Annuities
An annuity is a series of equal dollar payments coming
at the end of each time period for a specific
time period.
Pension funds, insurance obligations, and interest received from bonds are annuities.
Слайд 23Compound Annuities
A compound annuity involves depositing an equal sum of
money at the end of each year for a certain
number of years, allowing it to grow.
Constant periodic payments may be for an education, a new car, or any time you want to know how much your savings will have grown by some point in the future.
Слайд 24Compound Annuities
Example: You deposit $500 at the end of each
year for the next 5 years. If the bank pays
6% interest, how much will you have at the end of 5 years?
Future value of an annuity = annual payment x future value interest factor of an annuity.
Use Table 3.6, column i = 6%, row n = 5, the FVIFA is 5.637.
$500 x 5.637 = $2,818.50 at the end of 5 years.
Слайд 25Compound Annuities
Example: You need $10,000 for education in 8 years.
How much must you put away at the end of
each year at 6% interest to have the college money available?
You know the values of n, i, and FVn, but don’t know the PMT.
You must deposit $1010.41 at the end of each year at 6% interest to accumulate $10,000 at the end of 8 years.
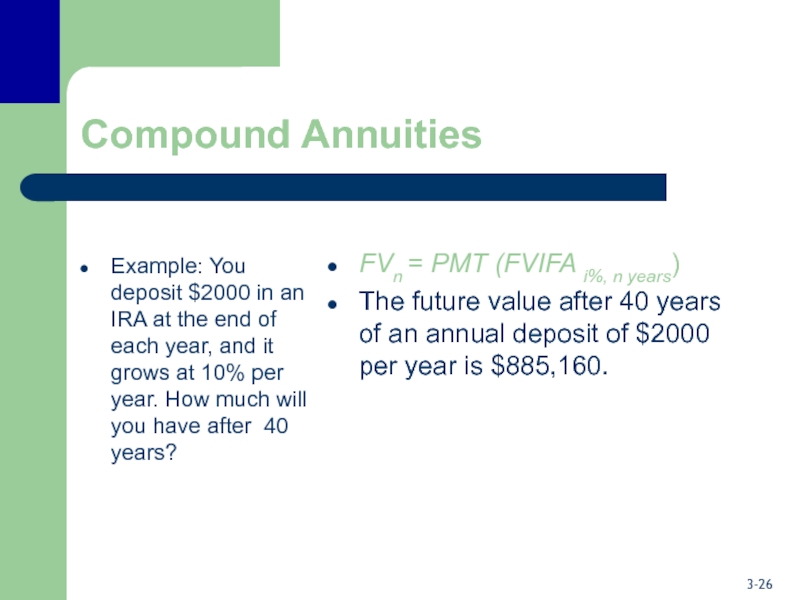
Слайд 26Compound Annuities
Example: You deposit $2000 in an IRA at the
end of each year, and it grows at 10% per
year. How much will you have after 40 years?
FVn = PMT (FVIFA i%, n years)
The future value after 40 years of an annual deposit of $2000 per year is $885,160.
Слайд 27Present Value of an Annuity
To compare the relative value of
annuities, you need to know the present value of each.
Use
the present-value interest factor for an annuity PFIVAi,n.
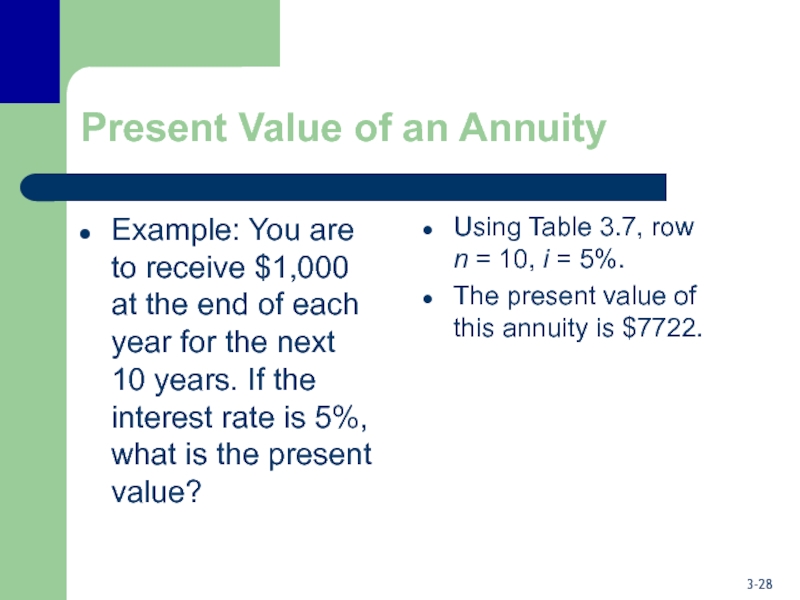
Слайд 28Present Value of an Annuity
Example: You are to receive $1,000
at the end of each year for the next 10
years. If the interest rate is 5%, what is the present value?
Using Table 3.7, row
n = 10, i = 5%.
The present value of this annuity is $7722.
Слайд 29Amortized Loans
Annuities usually involve paying off a loan in equal
installments over time.
Amortized loans are paid off this way.
Examples
include car loans and mortgages.
Слайд 30Amortized Loans
Example: You borrow $6000 at 15% interest to buy
a car and repay it in 4 equal payments at
the end of each of the next 4 years. What are the annual payments?
PV=$6000, i=15%, n=4.
Substituting into the equation the PMT would be $2101.58.
Слайд 31Perpetuities
A perpetuity is an annuity that continues forever.
Every year
this investment pays the same dollar amount and never stops
paying.
Present value of a perpetuity = payment/discount rate.
Слайд 32Perpetuities
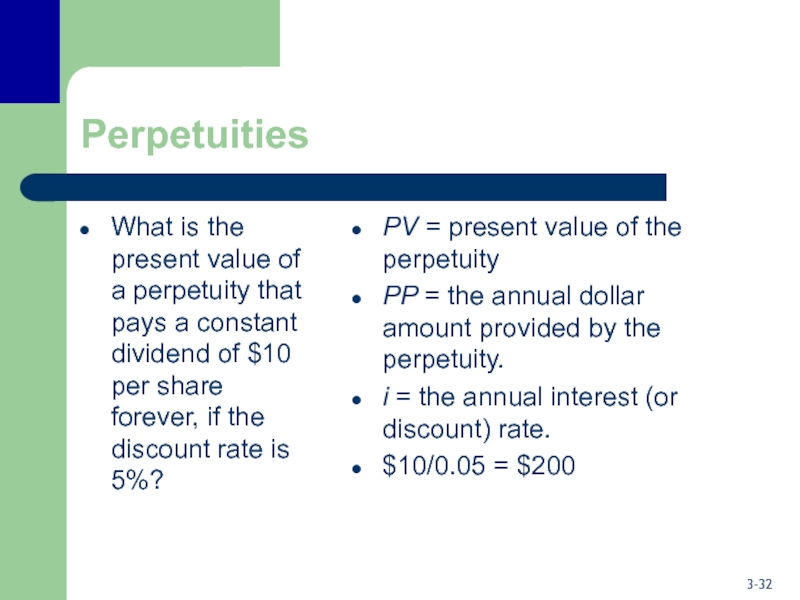
What is the present value of a perpetuity that pays
a constant dividend of $10 per share forever, if the
discount rate is 5%?
PV = present value of the perpetuity
PP = the annual dollar amount provided by the perpetuity.
i = the annual interest (or discount) rate.
$10/0.05 = $200







![Chapter 3 Present ValuePV = FVn[1/(1 + i)n]PV = present value of a Present ValuePV = FVn[1/(1 + i)n]PV = present value of a sum of money.FV = future value](/img/thumbs/73571e6aa859b14b0307c96d529ab0f4-800x.jpg)
![Chapter 3 Present ValueTables can be used to calculate the [1/(1+i)n] part of Present ValueTables can be used to calculate the [1/(1+i)n] part of the equation. This is the present-value](/img/thumbs/247744391c33d605839da56a6c27b753-800x.jpg)