Complex Numbers

has a real part and an imaginary part:
z = a

+ bi, where a and b are real numbers and i = √ – 1.
Examples of complex numbers:
1 + i, – 1 + 16i, 153 – i, 0.000002 + 5301.7i, – i
The last example given is a complex number with real part zero; this is called a “pure imaginary” number.

The entire set of real numbers is embedded within the complex numbers: z = a + 0i
The entire set of imaginary numbers is embedded within the complex numbers: z = 0 + bi
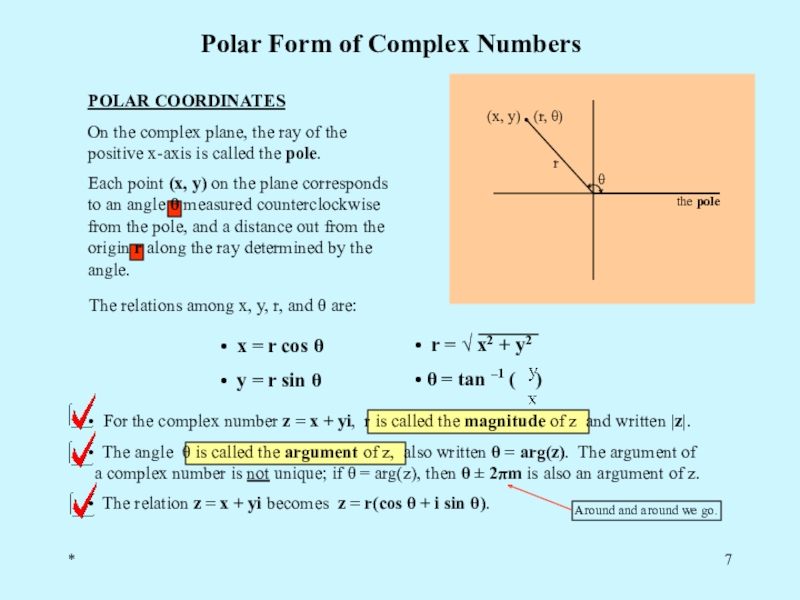
Any point (x,y) on the Cartesian plane corresponds to a complex number z = x + yi
(the Cartesian plane then is referred to as the “complex plane”)
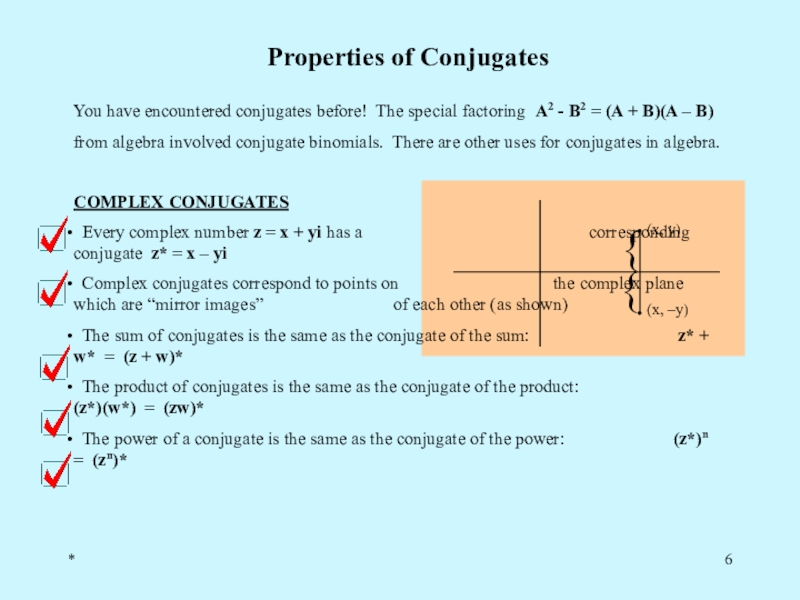
A complex number z = a + bi has another complex number associated with it, called its conjugate, z* = a – bi
(z and z* are conjugates of each other: z is the conjugate of z*)

and imaginary parts to imaginary parts:
(a + bi) + (c

+ di) = (a + c) + (b + d)i
Examples:
(16 + i) + (7 – 11i) = (16 + 7) + (1 – 11)i = 23 – 10i
(–2 – 5i) + (–3 + 7i) = (–2 + (–3)) + (–5 + 7)i = –5 + 2i

Subtraction
Just subtract real parts from real parts and imaginary parts from imaginary parts:
(a + bi) – (c + di) = (a – c) + (b – d)i
Examples:
(16 + i) – (7 – 11i) = (16 – 7) + (1 – (– 11))i
= (16 – 7) + (1 + 11)i = 9 + 12i
(–2 – 5i) – (–3 + 7i) = (–2 – (–3)) + (–5 – 7)i
= (–2 + 3) + (–5 – 7)i = 1 – 12i

distributive property:
(a + bi)(c + di) = (a + bi)(c)

+ (a + bi)(di)
= ac + bci + adi + bd(i2) = (ac – bd) + (ad + bc)i
Notice that we used the definition of i: it is the number which squared gives –1.
Examples:
(–2 – 5i)(–3 + 7i) = ((–2)(–3) – (–5)(7)) + ((–2)(7) + (–5)(–3))i
= (6 – (–35)) + (–14 + 15)i = 41 + i

(7 + ½ i)(–2 + i) = ((7)(–2) – (½)(1)) + ((7)(1) + (½)(–2))i
= (–14 – ½) + (7 – 1)i = – 29 + 6i

2

Properties of i
i0 = 1, i1 = i, i2 = –1, i3 = –i, i4 = 1, i5 = i, i6 = –1, etc.
i–1 = –i, i–2 = –1, i–3 = i, i–4 = 1, i–5 = –i, i–6 = –1, etc.

properties of complex conjugates (next slide):
a + bi (a + bi)(c

– di) (ac + bd) + (– ad + bc)i

First we multiply the fraction (a + bi) / (c + di) by one. But we choose the form of one to
be the complex conjugate of the denominator: (c – di) / (c – di). Then just multiply the
numerator factors and the denominator factors. This makes the denominator pure real,
taking advantage of the fact that (c + di)(c - di) = c2 + d2. Examples:
16 + i (16 + i)(–3 – 7i) (–48 + 7) + (–112 – 3)i –41 – 115i

3 – 13i (3 – 13i)(– 5i) – 65 – 15i – 5(13 + 3i) –13 – 3i

c + di (c + di)(c – di) c2 + d2

=

=

–3 + 7i (–3 + 7i)(–3 – 7i) 9 + 49 58

5i (5i)(– 5i) 25 25 5

=

=

=

=

=

=

=





A2 - B2 = (A + B)(A – B)
from algebra

involved conjugate binomials. There are other uses for conjugates in algebra.

COMPLEX CONJUGATES
Every complex number z = x + yi has a corresponding conjugate z* = x – yi
Complex conjugates correspond to points on the complex plane which are “mirror images” of each other (as shown)
The sum of conjugates is the same as the conjugate of the sum: z* + w* = (z + w)*
The product of conjugates is the same as the conjugate of the product: (z*)(w*) = (zw)*
The power of a conjugate is the same as the conjugate of the power: (z*)n = (zn)*

.

.

(x, y)

(x, –y)

{

{

ray of the positive x-axis is called the pole.
Each point

(x, y) on the plane corresponds to an angle θ measured counterclockwise from the pole, and a distance out from the origin r along the ray determined by the angle.

.

(x, y)

(r, θ)

r

θ

The relations among x, y, r, and θ are:

x = r cos θ
y = r sin θ

r = √ x2 + y2
θ = tan –1 ( )

For the complex number z = x + yi, r is called the magnitude of z and written |z|.
The angle θ is called the argument of z, also written θ = arg(z). The argument of a complex number is not unique; if θ = arg(z), then θ ± 2πm is also an argument of z.
The relation z = x + yi becomes z = r(cos θ + i sin θ).

the pole

Around and around we go.

= r1(cos θ1 + i sin θ1) r2(cos θ2 +

i sin θ2)
= r1r2(cos θ1 cos θ2 + i2 sin θ1 sin θ2 + i (cos θ1 sin θ2 + sin θ1 cos θ2))
= r1r2(cos θ1 cos θ2 – sin θ1 sin θ2 + i (cos θ1 sin θ2 + sin θ1 cos θ2))
= r1r2(cos [θ1 + θ2] + i sin [θ1 + θ2] )
In words, to multiply two complex numbers, simply multiply their magnitudes and add their arguments.

For division, such as z1/z2 = r1(cos θ1 + i sin θ1)/ r2(cos θ2 + i sin θ2), we need to take a look at the denominator:

r2(cos θ2 + i sin θ2) r2(cos θ2 + i sin θ2) r2(cos θ2 – i sin θ2)

r2(cos2 θ2 – i2 sin2 θ2)

r2(cos θ2 – i sin θ2)

1

=

cos θ2 – i sin θ2

=

=

r2–1(cos θ2 – i sin θ2)

a complex number we have just investigated,
division of complex numbers

has a simple formula, just like multiplication:
z1/z2 = r1(cos θ1 + i sin θ1)/ r2(cos θ2 + i sin θ2)
= r1 r2–1(cos [θ1 – θ2] + i sin [θ1 – θ2] )

NOTATION
Because of the algebraic form of the argument, cos θ + i sin θ, a complex number
z = r(cos θ + i sin θ) may be written as z = r(cis θ).
This shortened form makes the formulas for multiplication and division much nicer:

Multiplication of complex numbers: z1z2 = r1r2 cis [θ1 + θ2]
Division of complex numbers: z1/z2 = r1 r2–1 cis [θ1 – θ2]

and its conjugate.

3. Subtract 3 – 5i from its conjugate.

4.

(4x – yi) + (x + 3yi) =

5. (– 11 – 2i) – (– 9 – 4i) =

6. (4 + 3i)(4 – 3i) =

7. (4 + 3i)(3 + 4i) =

8. (6 + 3i) ÷ (1 – i) =

9. 1 1

10. (1 – i)(4 + 3i)

11. Show that √2 i√2
is a square root of i.

4 + 3i 4 – 3i

+

=

(2 + i)(1 + 2i)

=

2 2

+

( )

12. Two complex numbers are equal
if their real parts are equal and their
imaginary parts are equal.
Solve for a and b so that
( a + bi)(2 – 3i) = (2 + 3i) + (a + bi).