Разделы презентаций
- Разное
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
Lect 2
Содержание
- 1. Lect 2
- 2. Quiz 2 Absolute error and standard deviation are in the following relation:
- 3. Quiz 3
- 4. Course of lectures «Contemporary Physics: Part1»Lecture №2Motion in One Dimension.Motion in Two Dimensions.
- 5. Position, Velocity, and SpeedKinematics is the part
- 6. Position, Velocity, and SpeedThe displacement of a
- 7. Instantaneous Velocity and Speed The instantaneous speed of
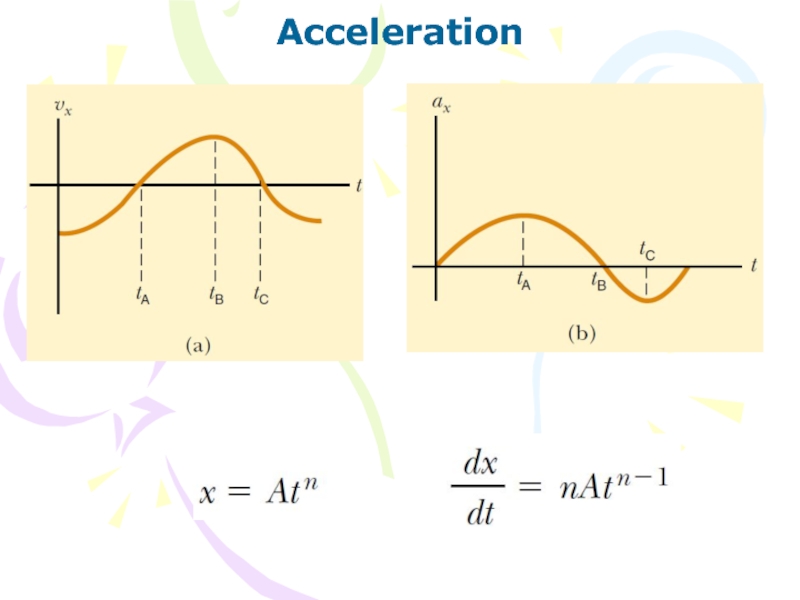
- 8. Acceleration(1.6) (1.7)The instantaneous acceleration equals the derivative of the velocity with respect to time.(1.8)
- 9. Acceleration
- 10. Motion Diagramsa) Motion diagram for a car
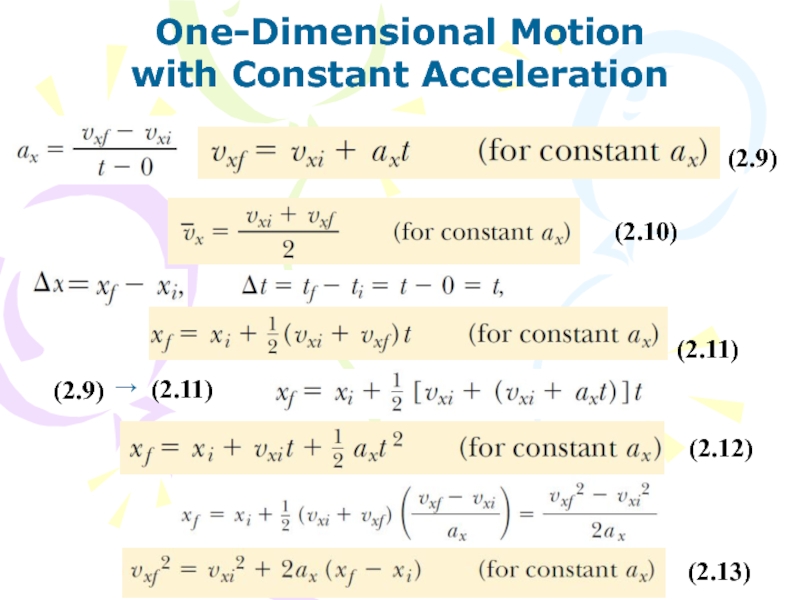
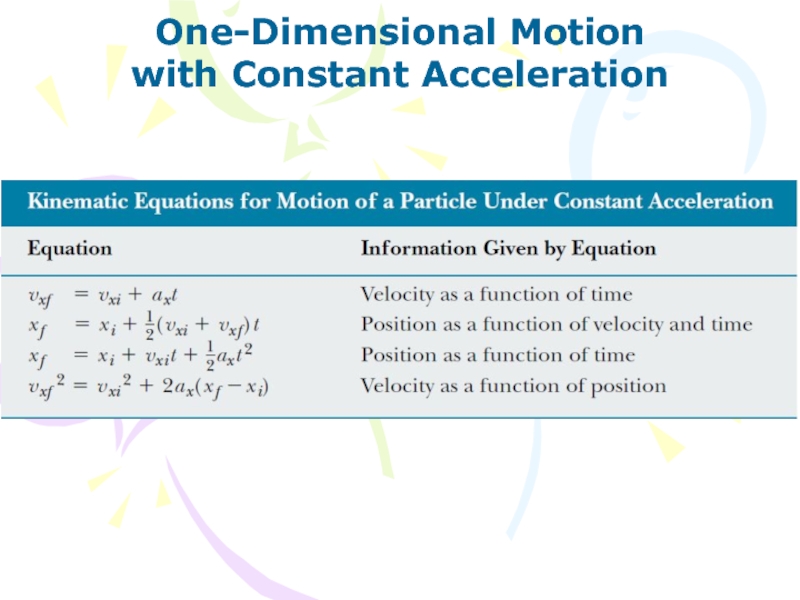
- 11. One-Dimensional Motion with Constant Acceleration
- 12. One-Dimensional Motion with Constant Acceleration (2.9)(2.10)(2.11)(2.12)(2.13)
- 13. One-Dimensional Motion with Constant Acceleration
- 14. is a displacement vector(2.14)
- 15. The average velocity v of an object
- 16. Слайд 16
- 17. (2.16)
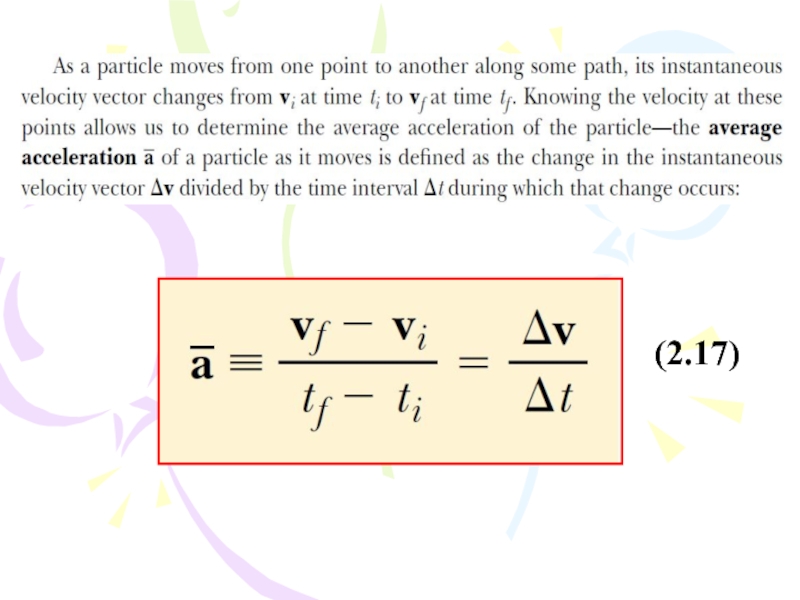
- 18. Average acceleration
- 19. (2.17)
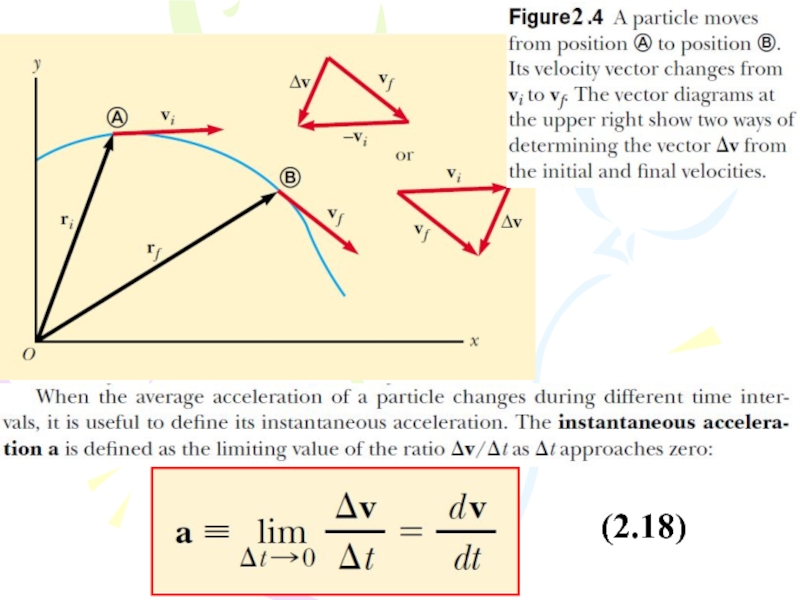
- 20. (2.18)
- 21. Two-Dimensional Motion with Constant AccelerationIf the position vector is known,(2.19)(2.20)
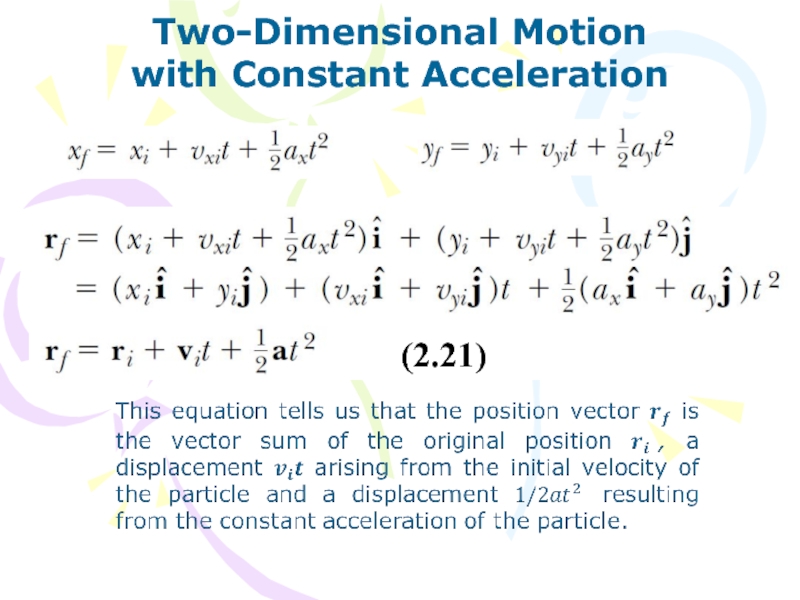
- 22. Two-Dimensional Motion with Constant Acceleration (2.21)
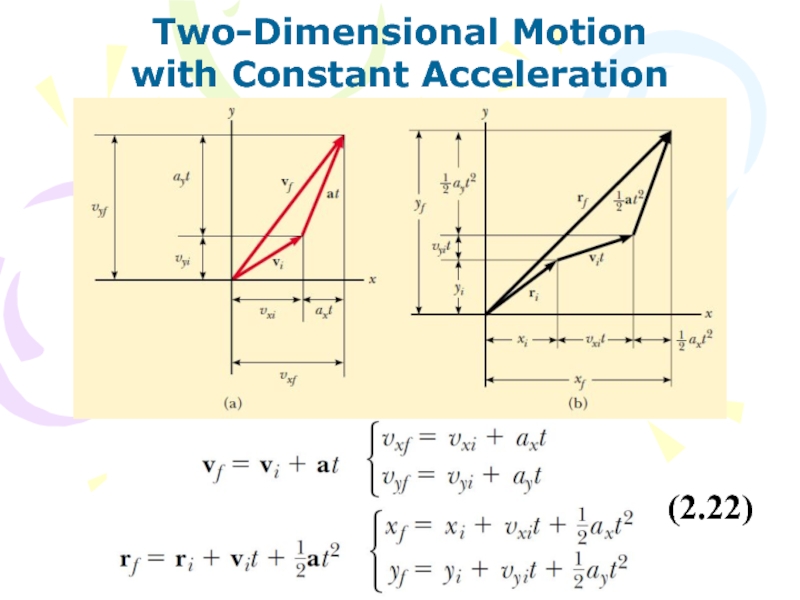
- 23. Two-Dimensional Motion with Constant Acceleration(2.22)
- 24. Projectile Motion(1) g is constant over the
- 25. Projectile Motion
- 26. Projectile MotionThe vector expression for the position
- 27. Even though an object moves at a
- 28. The acceleration vector in uniform circular motion
- 29. In many situations it is convenient to
- 30. Figure 2.6. The motion of a particle
- 31. The tangential acceleration component causes the change
- 32. Quick Quiz 1 If a car is
- 33. Скачать презентанцию
Слайды и текст этой презентации
Слайд 4Course of lectures «Contemporary Physics: Part1»
Lecture №2
Motion in One Dimension.
Motion
in Two Dimensions.
Слайд 5Position, Velocity, and Speed
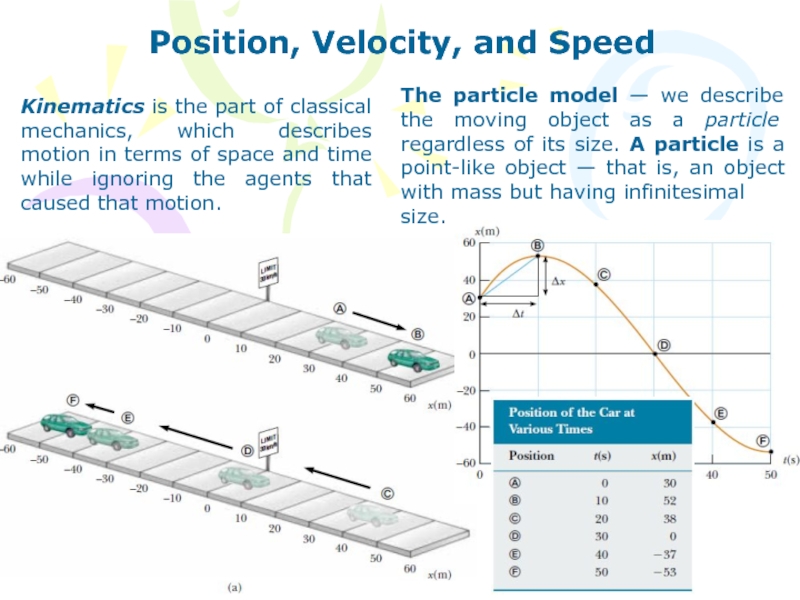
Kinematics is the part of classical mechanics,
which describes motion in terms of space and time while
ignoring the agents that caused that motion.The particle model — we describe the moving object as a particle regardless of its size. A particle is a point-like object — that is, an object with mass but having infinitesimal
size.
Слайд 6Position, Velocity, and Speed
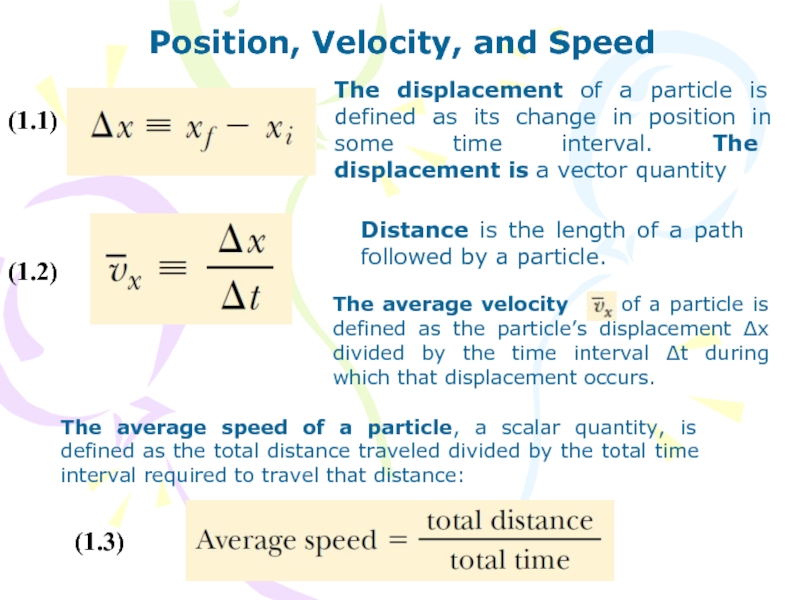
The displacement of a particle is defined
as its change in position in some time interval. The
displacement is a vector quantityDistance is the length of a path followed by a particle.
The average velocity of a particle is defined as the particle’s displacement Δx divided by the time interval Δt during which that displacement occurs.
The average speed of a particle, a scalar quantity, is defined as the total distance traveled divided by the total time interval required to travel that distance:
(1.1)
(1.2)
(1.3)
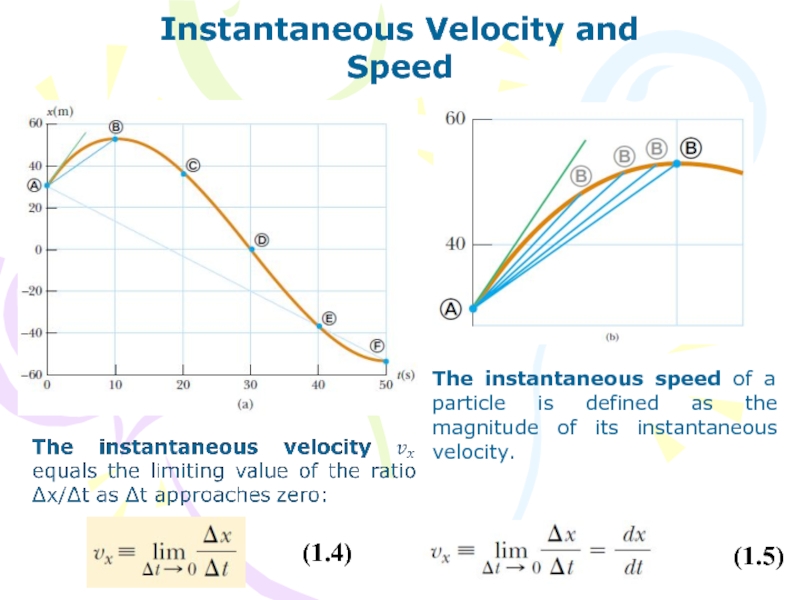
Слайд 7Instantaneous Velocity and Speed
The instantaneous speed of a particle is
defined as the magnitude of its instantaneous velocity.
(1.4)
(1.5)
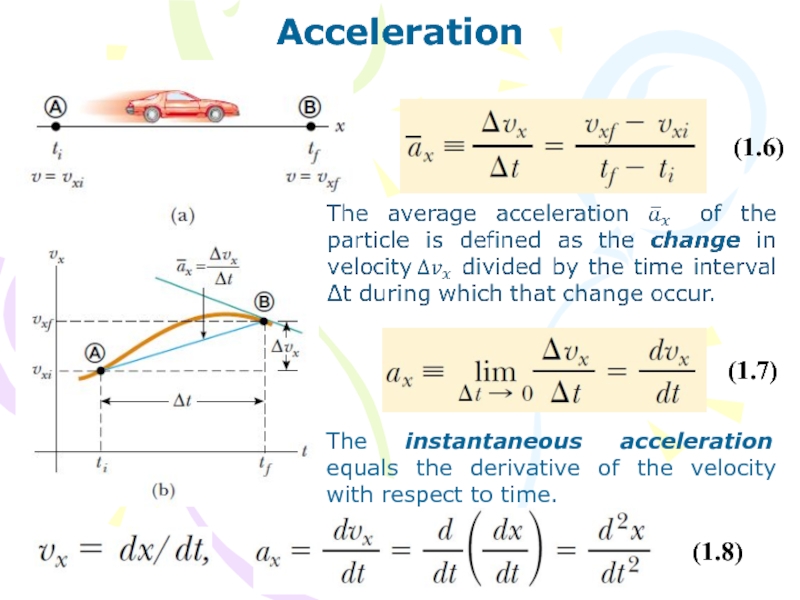
Слайд 8Acceleration
(1.6)
(1.7)
The instantaneous acceleration equals the derivative of the velocity with
respect to time.
(1.8)
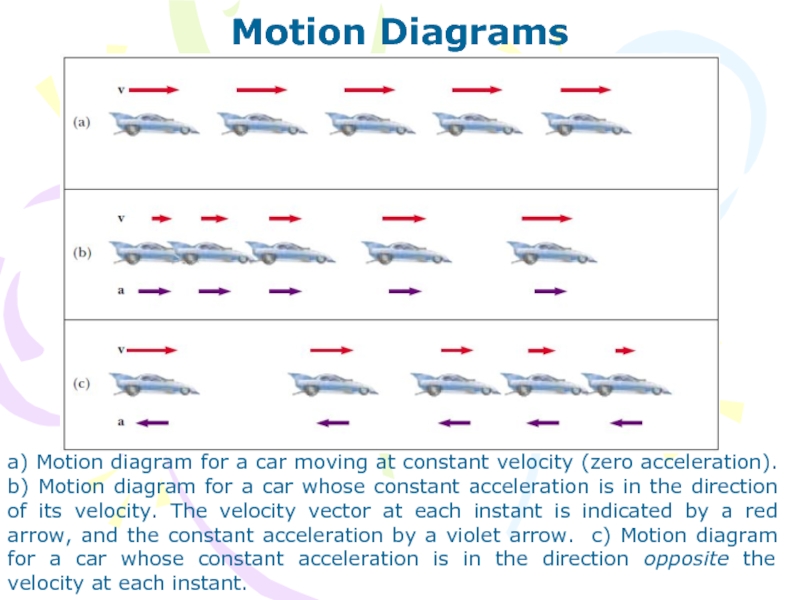
Слайд 10Motion Diagrams
a) Motion diagram for a car moving at constant
velocity (zero acceleration). b) Motion diagram for a car whose
constant acceleration is in the direction of its velocity. The velocity vector at each instant is indicated by a red arrow, and the constant acceleration by a violet arrow. c) Motion diagram for a car whose constant acceleration is in the direction opposite the velocity at each instant.Слайд 15The average velocity v of an object moving through a
displacement during a time interval (Δt) is described by the
formula(2.15)
Note that the average velocity between points is independent of the path taken.
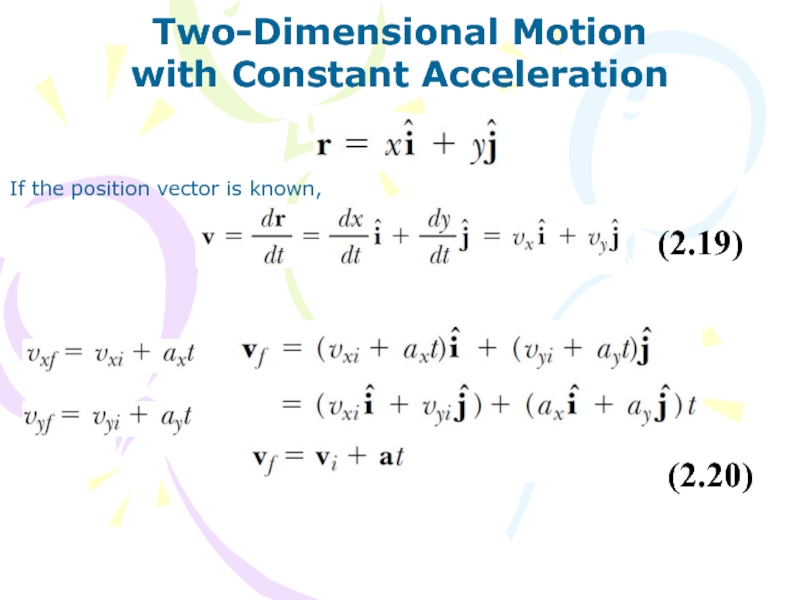
Слайд 21Two-Dimensional Motion with Constant Acceleration
If the position vector is known,
(2.19)
(2.20)
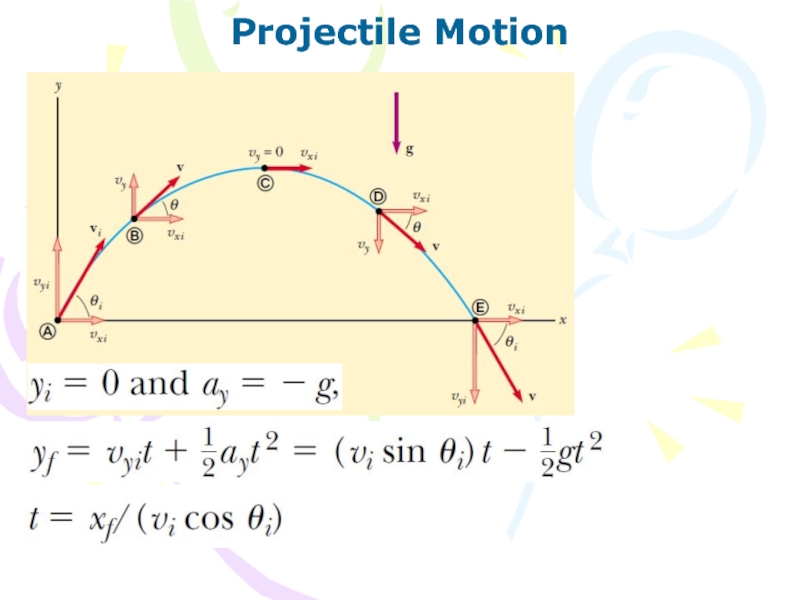
Слайд 24Projectile Motion
(1) g is constant over the range of motion
and is directed Downward
(2) the effect of air resistance is
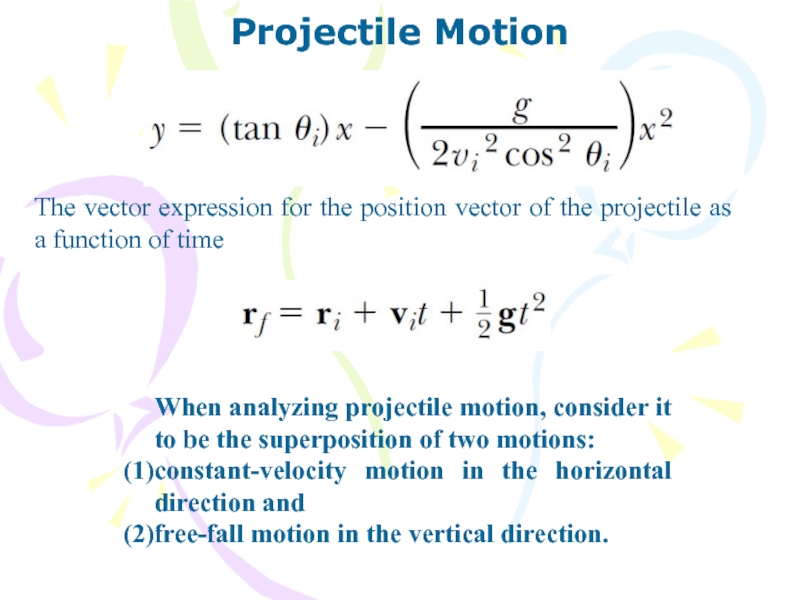
negligibleСлайд 26Projectile Motion
The vector expression for the position vector of the
projectile as a function of time
When analyzing projectile motion, consider
it to be the superposition of two motions:constant-velocity motion in the horizontal direction and
free-fall motion in the vertical direction.
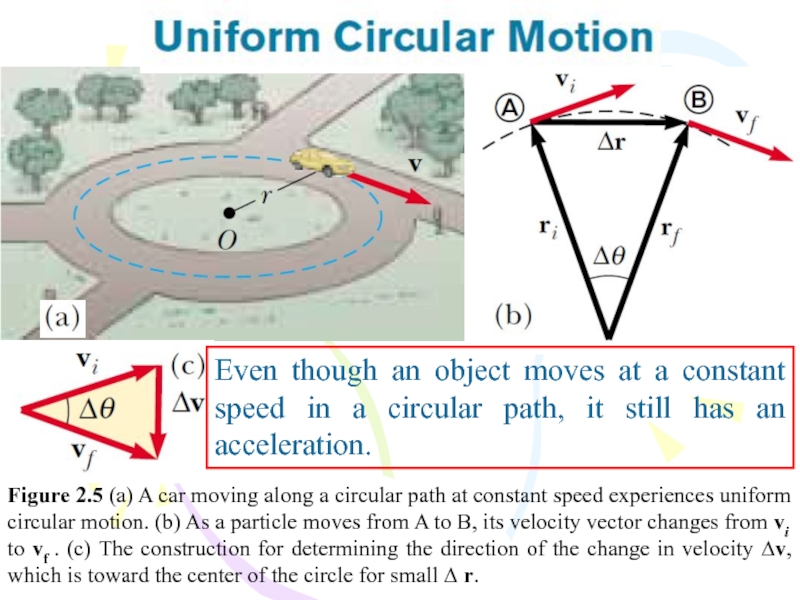
Слайд 27Even though an object moves at a constant speed in
a circular path, it still has an acceleration.
Figure 2.5 (a)
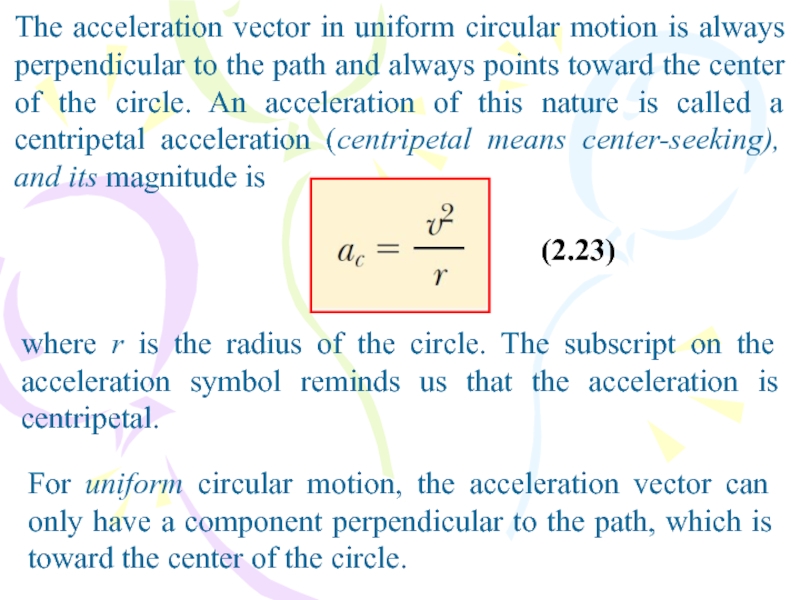
A car moving along a circular path at constant speed experiences uniform circular motion. (b) As a particle moves from A to B, its velocity vector changes from vi to vf . (c) The construction for determining the direction of the change in velocity ∆v, which is toward the center of the circle for small ∆ r.Слайд 28The acceleration vector in uniform circular motion is always perpendicular
to the path and always points toward the center of
the circle. An acceleration of this nature is called a centripetal acceleration (centripetal means center-seeking), and its magnitude is(2.23)
where r is the radius of the circle. The subscript on the acceleration symbol reminds us that the acceleration is centripetal.
For uniform circular motion, the acceleration vector can only have a component perpendicular to the path, which is toward the center of the circle.
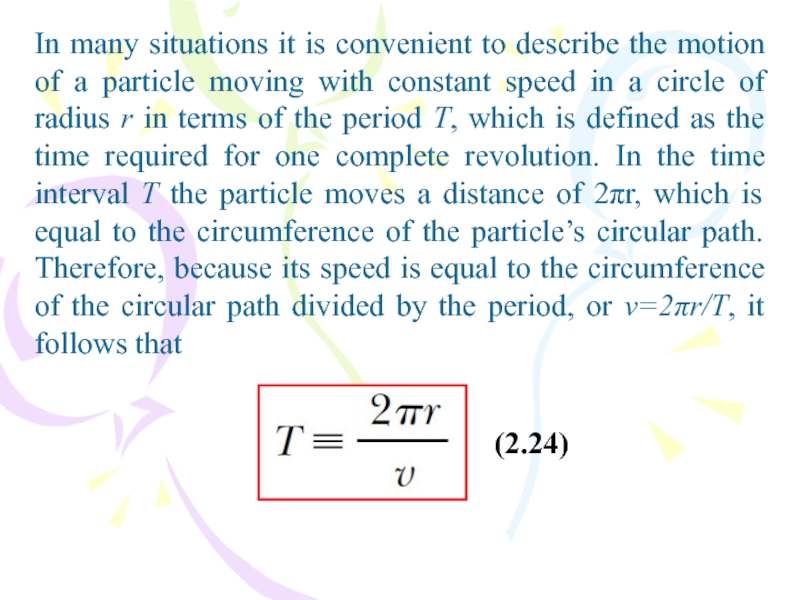
Слайд 29In many situations it is convenient to describe the motion
of a particle moving with constant speed in a circle
of radius r in terms of the period T, which is defined as the time required for one complete revolution. In the time interval T the particle moves a distance of 2πr, which is equal to the circumference of the particle’s circular path. Therefore, because its speed is equal to the circumference of the circular path divided by the period, or v=2πr/T, it follows that(2.24)
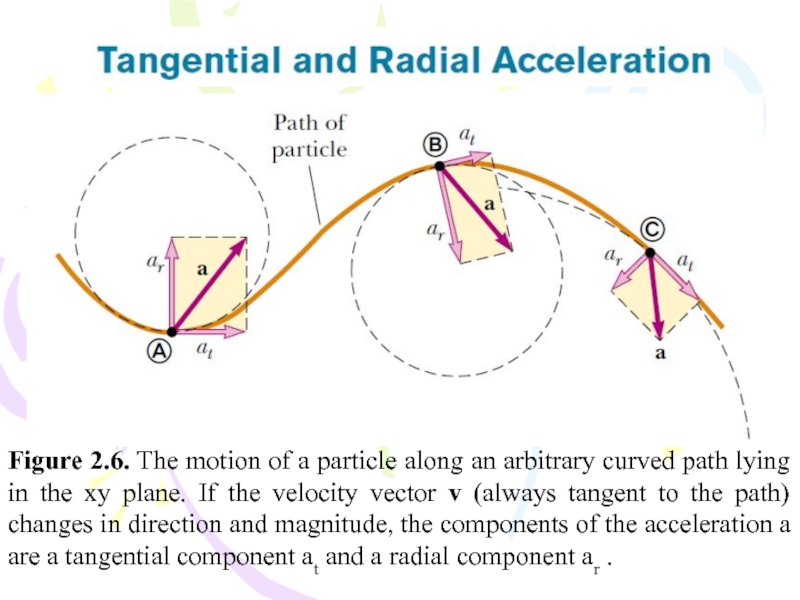
Слайд 30Figure 2.6. The motion of a particle along an arbitrary
curved path lying in the xy plane. If the velocity
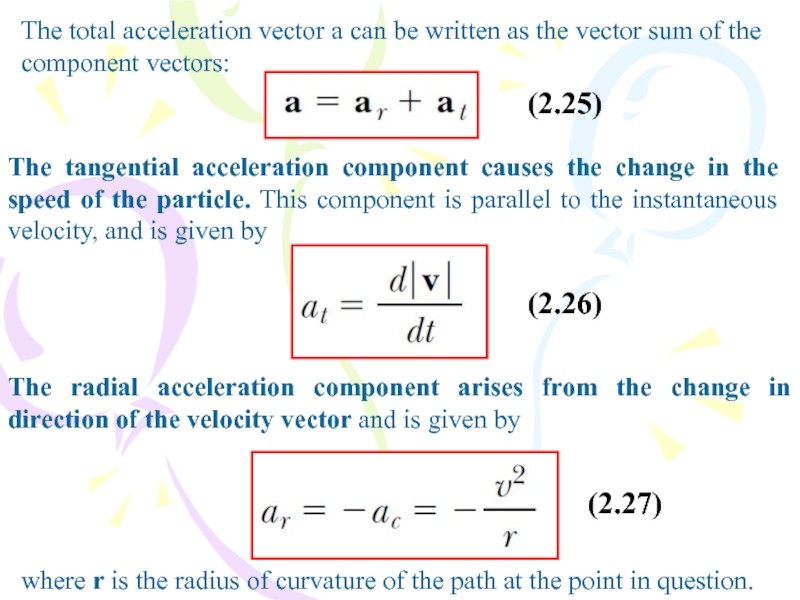
vector v (always tangent to the path) changes in direction and magnitude, the components of the acceleration a are a tangential component at and a radial component ar .Слайд 31The tangential acceleration component causes the change in the speed
of the particle. This component is parallel to the instantaneous
velocity, and is given byThe radial acceleration component arises from the change in direction of the velocity vector and is given by
where r is the radius of curvature of the path at the point in question.
The total acceleration vector a can be written as the vector sum of the component vectors:
(2.27)
(2.26)
(2.25)
Слайд 32Quick Quiz 1 If a car is traveling eastward and
slowing down, what is the direction of the force on
the car that causes it to slow down? (a) eastward (b) westward (c) neither of these.Quick Quiz 2 A ball is thrown upward. While the ball is in free fall, does its acceleration (a) increase (b) decrease (c) increase and then decrease (d) decrease and then increase (e) remain constant?
Quick Quiz 3 After a ball is thrown upward and is in the air, its speed (a) increases (b) decreases (c) increases and then decreases (d) decreases and then increases (e) remains the same.





























![АВТОМАТИЗАЦ ИЯ ЗВУКА [ Р ] В СЛ ОГ АХ, СЛОВАХ, ПРЕДЛОЖЕ Н И ЯХ](/img/tmb/7/629823/44b5dc2a0928b26438e7fc0460d4ba45-800x.jpg)

