Разделы презентаций
- Разное
- Английский язык
- Астрономия
- Алгебра
- Биология
- География
- Геометрия
- Детские презентации
- Информатика
- История
- Литература
- Математика
- Медицина
- Менеджмент
- Музыка
- МХК
- Немецкий язык
- ОБЖ
- Обществознание
- Окружающий мир
- Педагогика
- Русский язык
- Технология
- Физика
- Философия
- Химия
- Шаблоны, картинки для презентаций
- Экология
- Экономика
- Юриспруденция
03 Series
Содержание
- 1. 03 Series
- 2. Today’s ObjectivesDefinition of an arithmetic progression and the nth termSum of an arithmetic progression
- 3. Arithmetic Progressions (APs)An arithmetic progression is when
- 4. Arithmetic Progressions (APs)So the general formula for
- 5. AP Example 1The 8th term of an
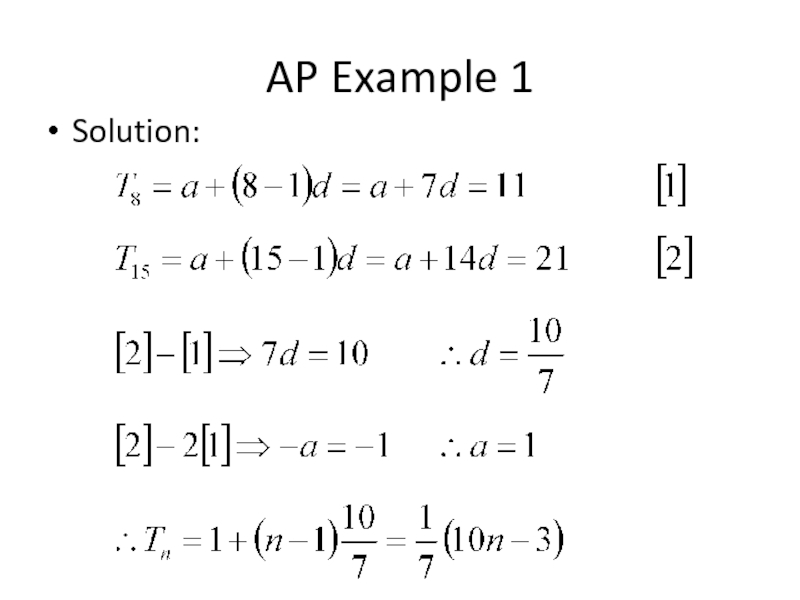
- 6. AP Example 1Solution:
- 7. AP Example 2The nth term of an
- 8. AP Example 2Solution:
- 9. AP Example 3An AP with all positive
- 10. AP Example 3i) Solution:All terms are positive so d > 0.
- 11. AP Example 3ii) Solution:Tn is a whole
- 12. Sum of an APConsidering the sum of
- 13. Sum of an APSo now consider the
- 14. Sum of an APSimplified we have: Hence
- 15. Sum of an APAlso, since the last
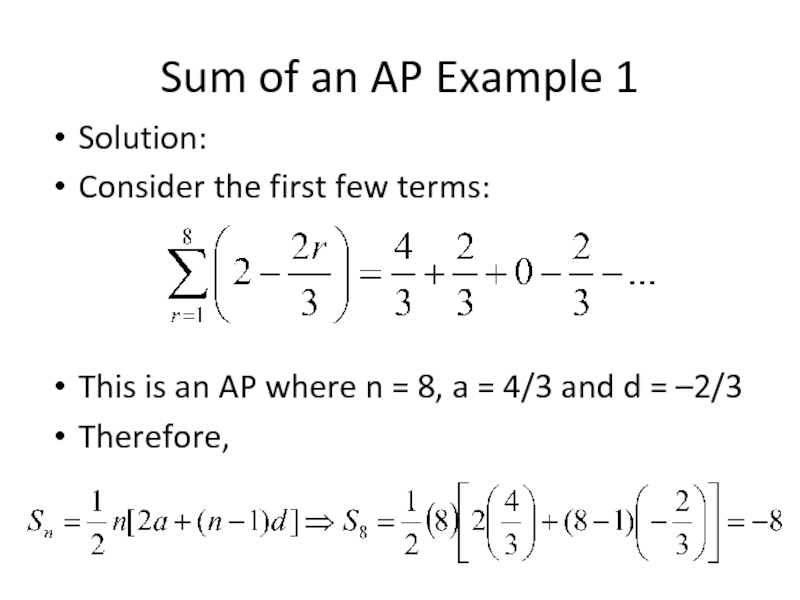
- 16. Sum of an AP Example 1EvaluateRather than
- 17. Sum of an AP Example 1Solution:Consider the
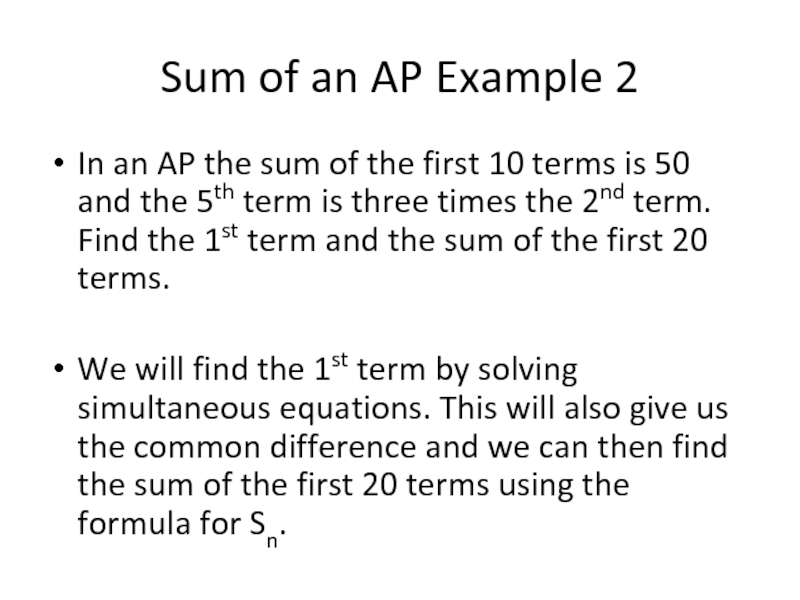
- 18. Sum of an AP Example 2In an
- 19. Sum of an AP Example 2Solution:
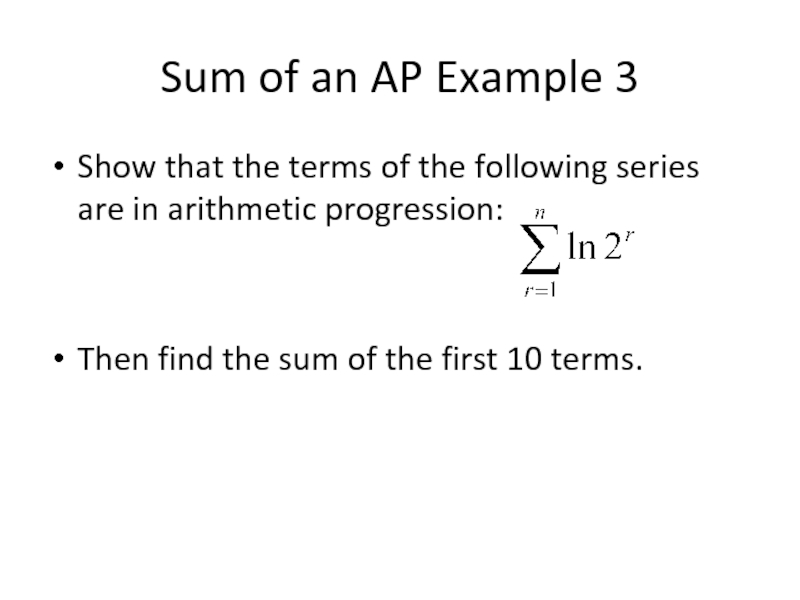
- 20. Sum of an AP Example 3Show that
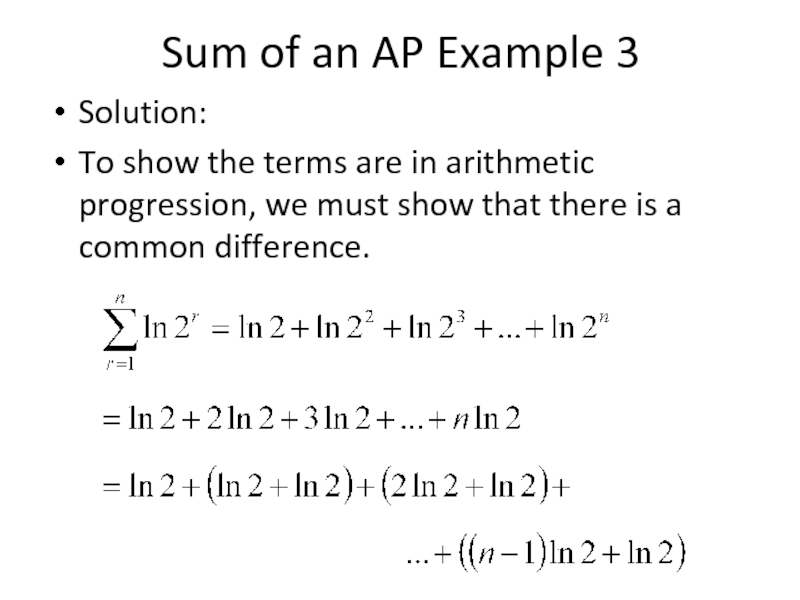
- 21. Sum of an AP Example 3Solution:To show
- 22. Sum of an AP Example 3There is
- 23. Sum of an AP Example 4The sum
- 24. Sum of an AP Example 4Solution: Finding the fourth term:
- 25. Sum of an AP Example 4Solution: Show
- 26. Sum of an AP Example 5Find the
- 27. Sum of an AP Example 5Solution:
- 28. Sum of an AP Example 6Find three
- 29. Sum of an AP Example 6Solution:Let the
- 30. Sum of an AP Example 6Solving [1]
- 31. In summaryAn arithmetic progression (AP) is a
- 32. Скачать презентанцию
Today’s ObjectivesDefinition of an arithmetic progression and the nth termSum of an arithmetic progression
Слайды и текст этой презентации
Слайд 2Today’s Objectives
Definition of an arithmetic progression and the nth term
Sum
of an arithmetic progression
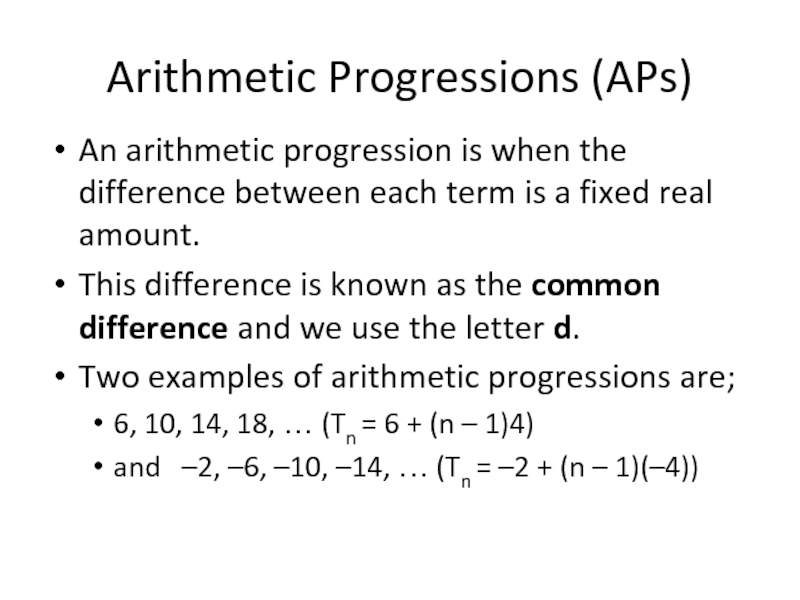
Слайд 3Arithmetic Progressions (APs)
An arithmetic progression is when the difference between
each term is a fixed real amount.
This difference is
known as the common difference and we use the letter d.Two examples of arithmetic progressions are;
6, 10, 14, 18, … (Tn = 6 + (n – 1)4)
and –2, –6, –10, –14, … (Tn = –2 + (n – 1)(–4))
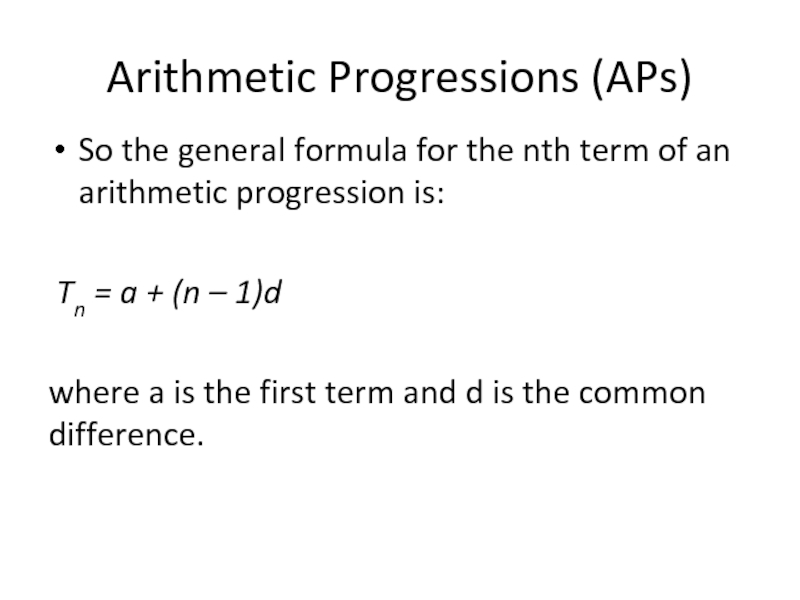
Слайд 4Arithmetic Progressions (APs)
So the general formula for the nth term
of an arithmetic progression is:
Tn = a +
(n – 1)d where a is the first term and d is the common difference.
Слайд 5AP Example 1
The 8th term of an AP is 11
and the 15th term is 21. What is the common
difference and the nth term?To answer this we will use the formula for the nth term, Tn = a + (n – 1)d, to find the common difference d and the first term a.
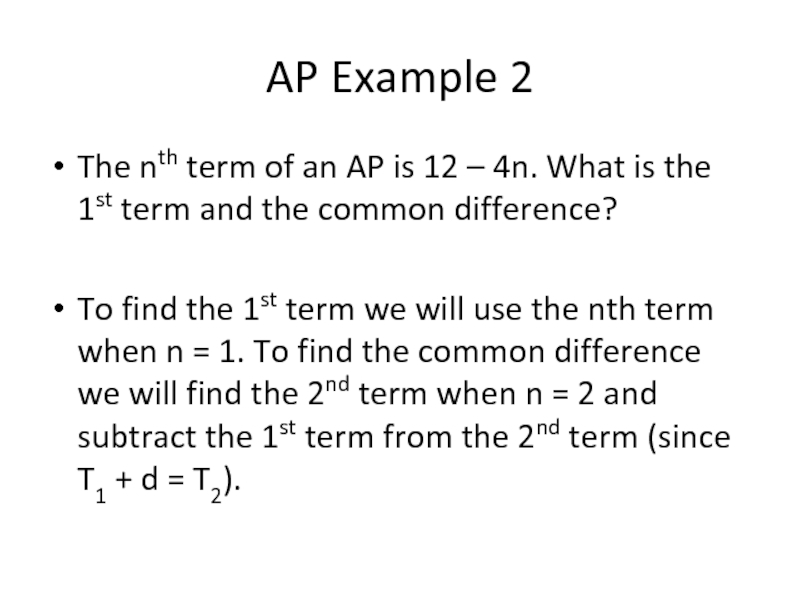
Слайд 7AP Example 2
The nth term of an AP is 12
– 4n. What is the 1st term and the common
difference?To find the 1st term we will use the nth term when n = 1. To find the common difference we will find the 2nd term when n = 2 and subtract the 1st term from the 2nd term (since T1 + d = T2).
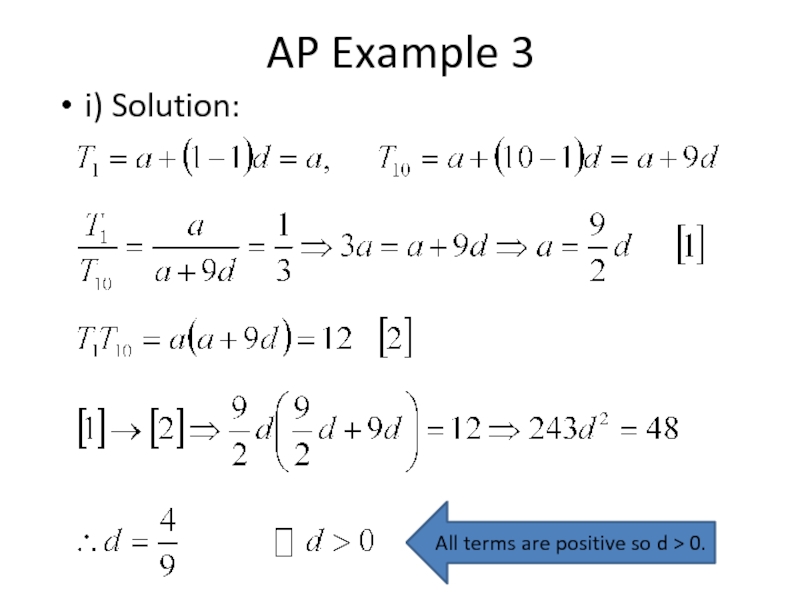
Слайд 9AP Example 3
An AP with all positive terms, is such
that T1T10 = 12 and T1/T10 = 1/3.
i) Find
the common difference, ii) Find the value of the next term after the 10th term which is a whole number.
i) To find the common difference we will use the nth term , Tn = a + (n – 1)d, to form simultaneous equations and solve for a and d.
ii) We must choose a value of n (n>10) to resolve any fractions in Tn.
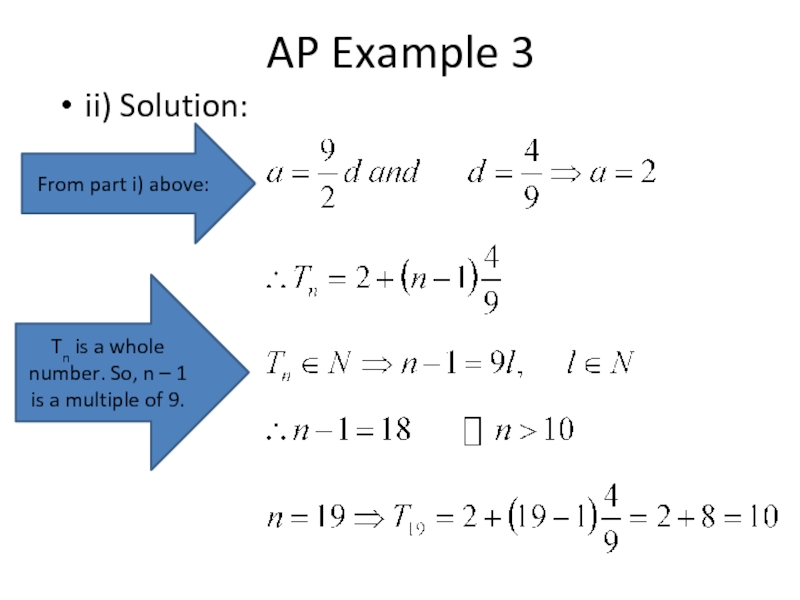
Слайд 11AP Example 3
ii) Solution:
Tn is a whole number. So, n
– 1 is a multiple of 9.
From part i) above:
Слайд 12Sum of an AP
Considering the sum of the first n
terms of the sequence (i.e. the series), where a is
the first term, d is the common difference and l is the last term:But this is rather long.
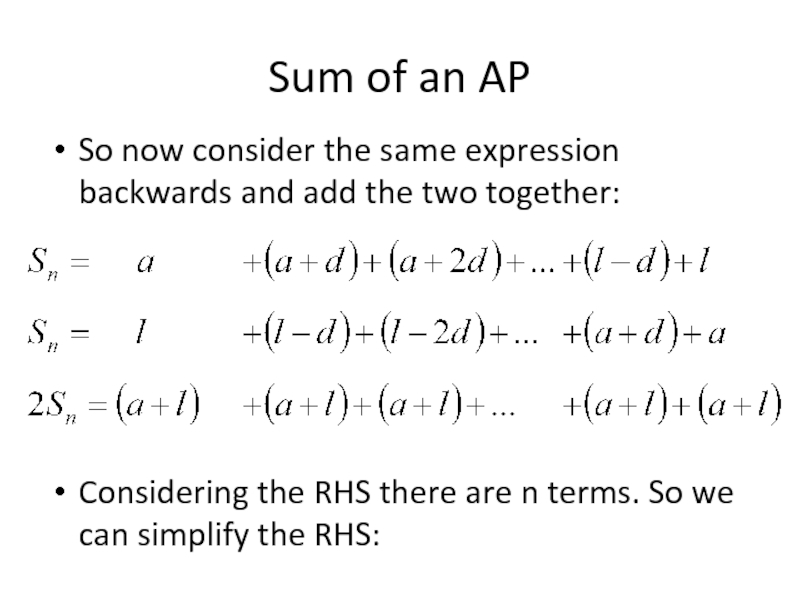
Слайд 13Sum of an AP
So now consider the same expression backwards
and add the two together:
Considering the RHS there are
n terms. So we can simplify the RHS:
Слайд 14Sum of an AP
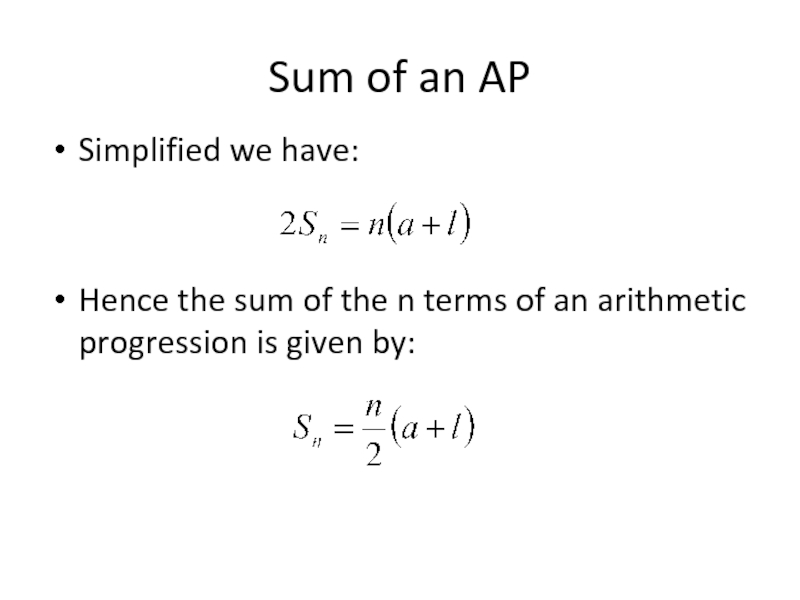
Simplified we have:
Hence the sum of
the n terms of an arithmetic progression is given by:
Слайд 15Sum of an AP
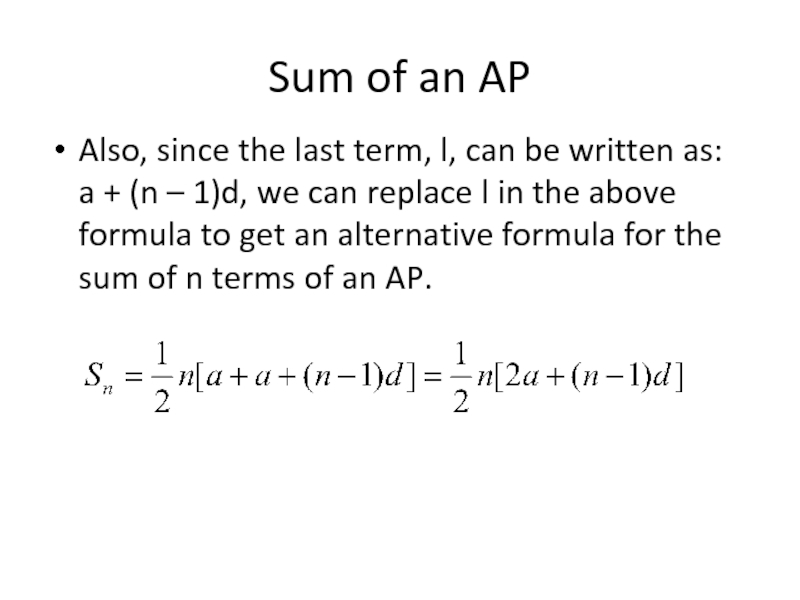
Also, since the last term, l, can
be written as: a + (n – 1)d, we can
replace l in the above formula to get an alternative formula for the sum of n terms of an AP.
Слайд 16Sum of an AP Example 1
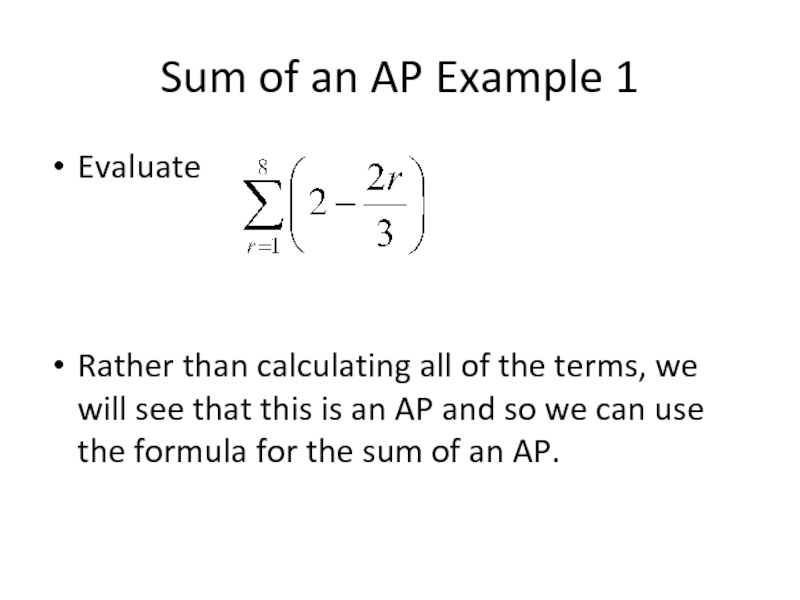
Evaluate
Rather than calculating all of
the terms, we will see that this is an AP
and so we can use the formula for the sum of an AP.
Слайд 17Sum of an AP Example 1
Solution:
Consider the first few terms:
This
is an AP where n = 8, a = 4/3
and d = –2/3Therefore,
Слайд 18Sum of an AP Example 2
In an AP the sum
of the first 10 terms is 50 and the 5th
term is three times the 2nd term. Find the 1st term and the sum of the first 20 terms.We will find the 1st term by solving simultaneous equations. This will also give us the common difference and we can then find the sum of the first 20 terms using the formula for Sn.
Слайд 20Sum of an AP Example 3
Show that the terms of
the following series are in arithmetic progression:
Then find the sum
of the first 10 terms.
Слайд 21Sum of an AP Example 3
Solution:
To show the terms are
in arithmetic progression, we must show that there is a
common difference.
Слайд 22Sum of an AP Example 3
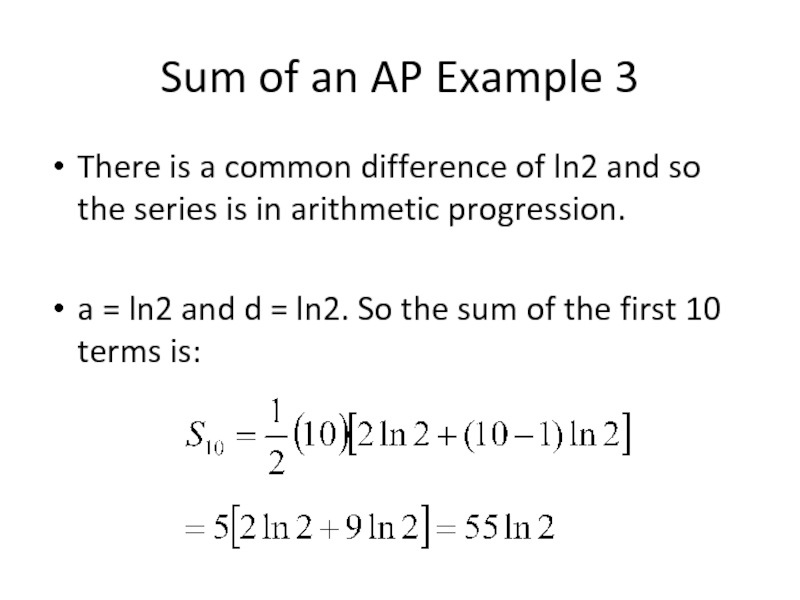
There is a common difference
of ln2 and so the series is in arithmetic progression.
a
= ln2 and d = ln2. So the sum of the first 10 terms is:
Слайд 23Sum of an AP Example 4
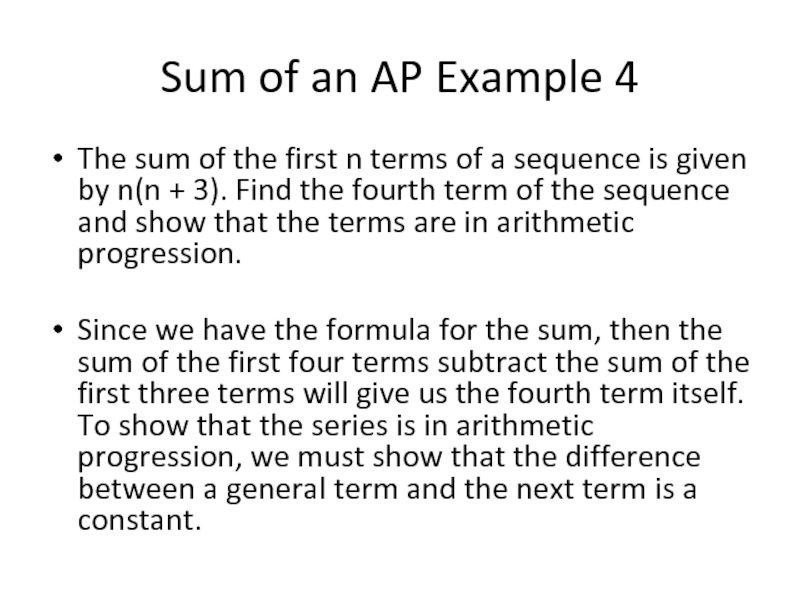
The sum of the first
n terms of a sequence is given by n(n +
3). Find the fourth term of the sequence and show that the terms are in arithmetic progression.Since we have the formula for the sum, then the sum of the first four terms subtract the sum of the first three terms will give us the fourth term itself. To show that the series is in arithmetic progression, we must show that the difference between a general term and the next term is a constant.
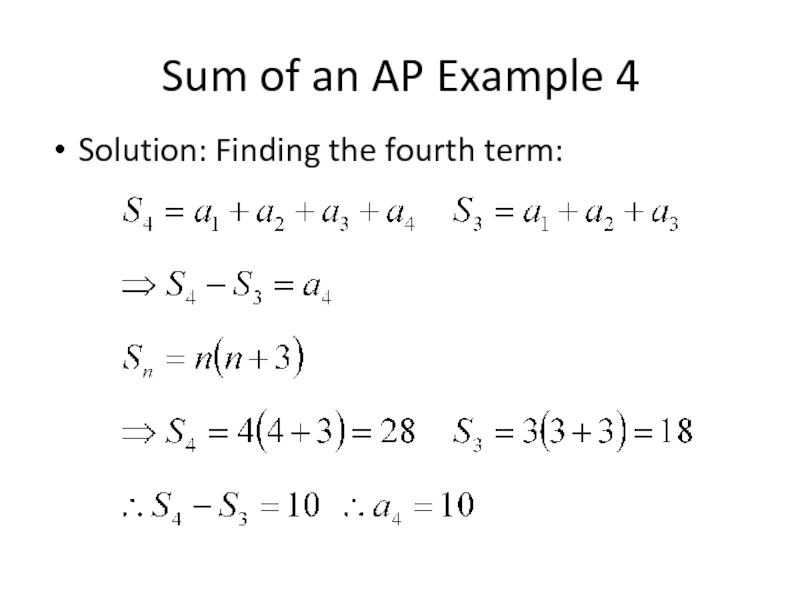
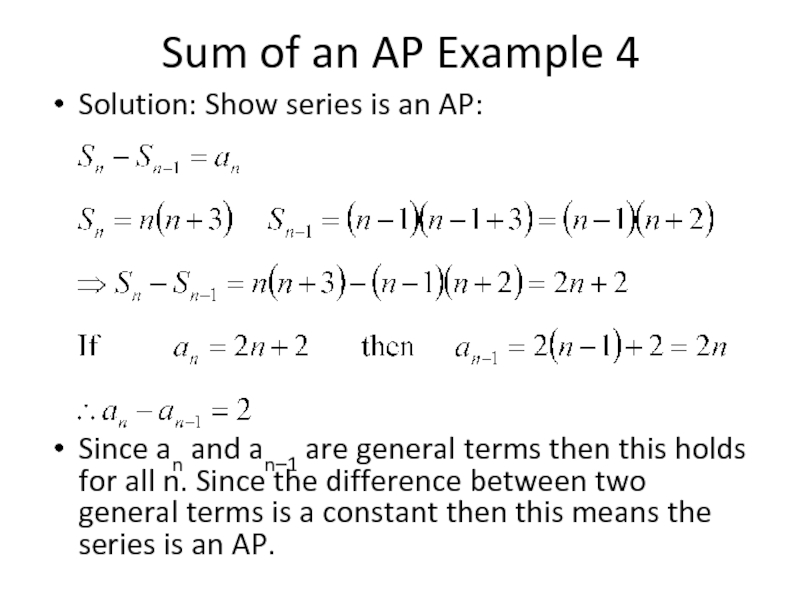
Слайд 25Sum of an AP Example 4
Solution: Show series is an
AP:
Since an and an–1 are general terms then this holds
for all n. Since the difference between two general terms is a constant then this means the series is an AP.
Слайд 26Sum of an AP Example 5
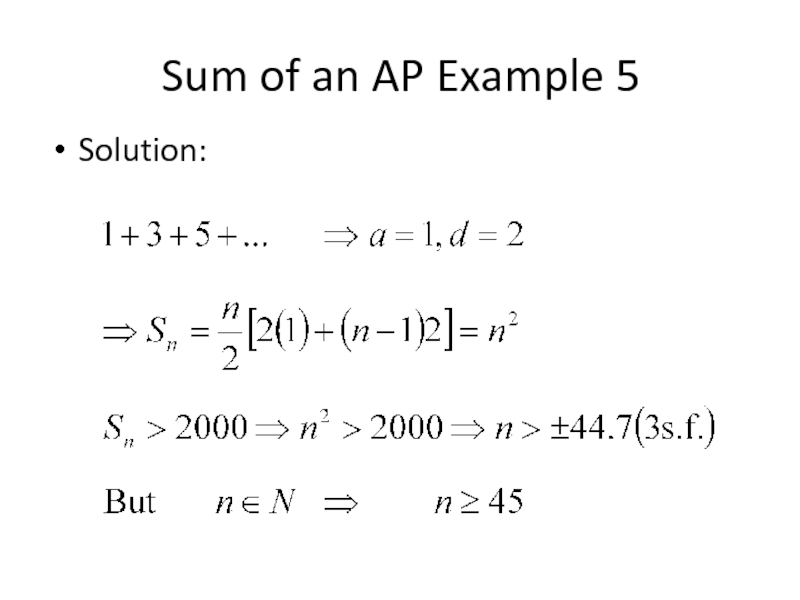
Find the least number of
terms of the AP 1 + 3 + 5 +
… that are required to make a sum exceeding 2000.We need to establish the first term and the common difference to solve the resulting inequality in n, Sn > 2000.
Слайд 28Sum of an AP Example 6
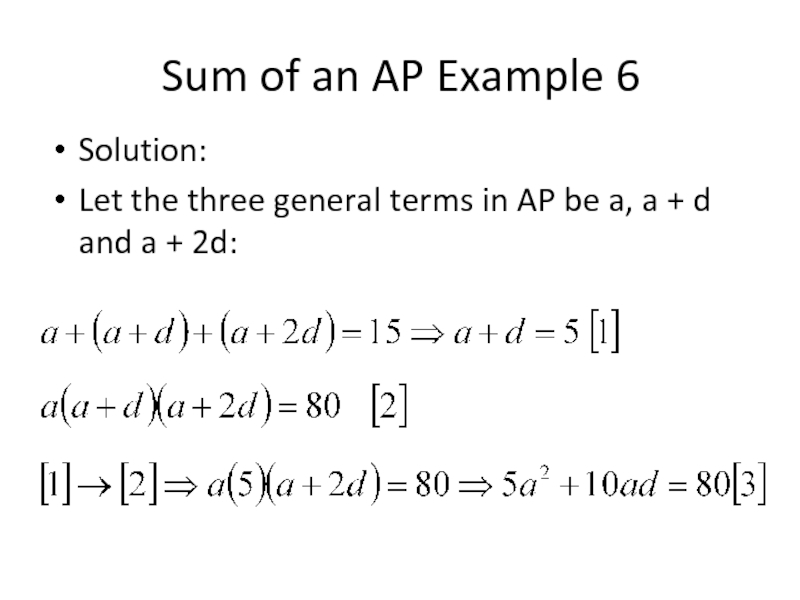
Find three numbers in AP
whose sum is 15 and whose product is 80.
We need
to consider three general terms in AP and then form simultaneous equations using their sum and their product.
Слайд 30Sum of an AP Example 6
Solving [1] and [3] gives
two solutions:
a = 8 and d = –3
a =
2 and d = 3Substituting either of these pairs into a, a + d and a + 2d gives the same 3 numbers.
Therefore the three numbers in AP are 2, 5 and 8.
![Sum of an AP Example 6Solving [1] and [3] gives two solutions:a = 8 and d =](/img/thumbs/c6d54b525a24bf9502a3a61e7e85a88e-800x.jpg "03 Series Sum of an AP Example 6Solving [1] and [3] gives two")
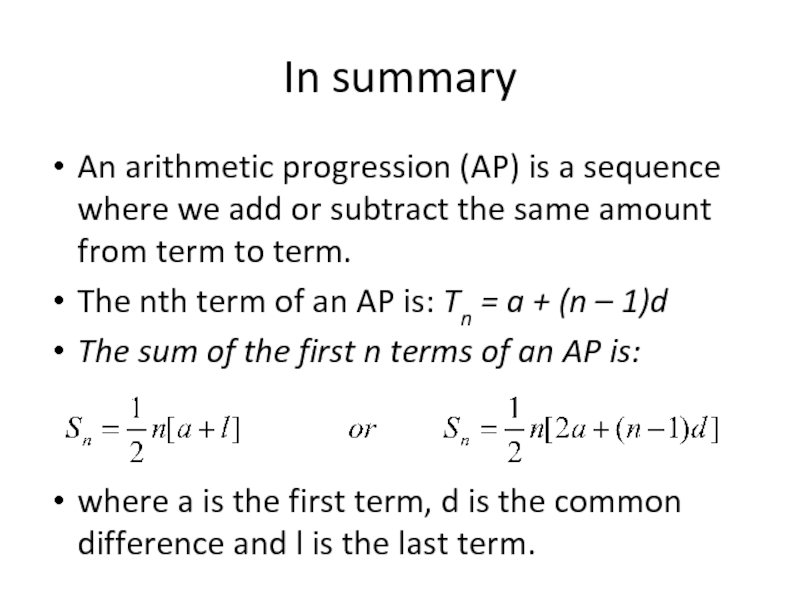
Слайд 31In summary
An arithmetic progression (AP) is a sequence where we
add or subtract the same amount from term to term.
The
nth term of an AP is: Tn = a + (n – 1)dThe sum of the first n terms of an AP is:
where a is the first term, d is the common difference and l is the last term.
